Saturday Quiz – February 19, 2011 – answers and discussion

Here are the answers with discussion for yesterday’s quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of modern monetary theory (MMT) and its application to macroeconomic thinking. Comments as usual welcome, especially if I have made an error.

Question 1:

If a sovereign national government runs a balanced budget (on average) over a business cycle, then the average private domestic deficit (surplus) will always be exactly equal to the average external deficit (surplus) over the same cycle.

The answer is True.

Note that this question begs the question as to how the economy might get into this situation that I have described using the sectoral balances framework. But whatever behavioural forces were at play, the sectoral balances all have to sum to zero. Once you understand that, then deduction leads to the correct answer.

To refresh your memory the balances are derived as follows. The basic income-expenditure model in macroeconomics can be viewed in (at least) two ways: (a) from the perspective of the sources of spending; and (b) from the perspective of the uses of the income produced. Bringing these two perspectives (of the same thing) together generates the sectoral balances.

From the sources perspective we write:

GDP = C + I + G + (X – M)

which says that total national income (GDP) is the sum of total final consumption spending (C), total private investment (I), total government spending (G) and net exports (X – M).

From the uses perspective, national income (GDP) can be used for:

GDP = C + S + T

which says that GDP (income) ultimately comes back to households who consume (C), save (S) or pay taxes (T) with it once all the distributions are made.

Equating these two perspectives we get:

C + S + T = GDP = C + I + G + (X – M)

So after simplification (but obeying the equation) we get the sectoral balances view of the national accounts.

(I – S) + (G – T) + (X – M) = 0

That is the three balances have to sum to zero. The sectoral balances derived are:

• The private domestic balance (I – S) – positive if in deficit, negative if in surplus.
• The Budget Deficit (G – T) – negative if in surplus, positive if in deficit.
• The Current Account balance (X – M) – positive if in surplus, negative if in deficit.

These balances are usually expressed as a per cent of GDP but that doesn’t alter the accounting rules that they sum to zero, it just means the balance to GDP ratios sum to zero.

A simplification is to add (I – S) + (X – M) and call it the non-government sector. Then you get the basic result that the government balance equals exactly $-for-$ (absolutely or as a per cent of GDP) the non-government balance (the sum of the private domestic and external balances).

This is also a basic rule derived from the national accounts and has to apply at all times.

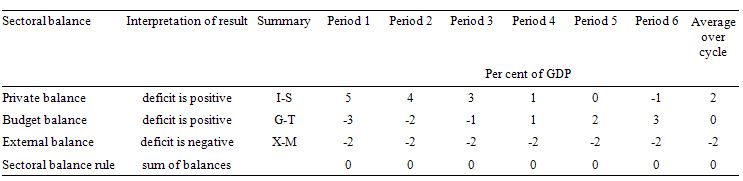
To help us answer the specific question posed, the following Table shows a stylised business cycle with some simplifications. The economy is running a surplus in the first three periods (but declining) and then increasing deficits. Over the entire cycle the balanced budget rule would be achieved as the budget balances average to zero. So the deficits are covered by fully offsetting surpluses over the cycle.

The simplification is the constant external deficit (that is, no cyclical sensitivity) of 2 per cent of GDP over the entire cycle. You can then see what the private domestic balance is doing clearly. When the budget balance is in surplus, the private balance is in deficit. The larger the budget surplus the larger the private deficit for a given external deficit.

As the budget moves into deficit, the private domestic balance approaches balance and then finally in Period 6, the budget deficit is large enough (3 per cent of GDP) to offset the demand-draining external deficit (2 per cent of GDP) and so the private domestic sector can save overall. The budget deficits are underpinning spending and allowing income growth to be sufficient to generate savings greater than investment in the private domestic sector.

On average over the cycle, under these conditions (balanced public budget) the private domestic deficit exactly equals the external deficit. As a result over the course of the business cycle, the private domestic sector becomes increasingly indebted.

The following blogs may be of further interest to you:

• Barnaby, better to walk before we run
• Stock-flow consistent macro models
• Norway and sectoral balances
• The OECD is at it again!

Question 2:

Excessive real wages growth can cause unemployment.

The answer is True.

In this blog – What causes mass unemployment? – I outline the way aggregate demand failures causes of mass unemployment and use a simple two person economy to demonstrate the point.

I also presented the famous Keynes versus the Classics debate about the role of real wage cuts in stimulating employment that was well rehearsed during the Great Depression.

The debate was multi-dimensioned but the role of wage flexibility was a key aspect. In the classical model of employment determination, which remains the basis of mainstream textbook analysis, cuts in the nominal wage will increase employment because it is considered they will reduce the real wage.

The mainstream textbook model assumes that economies produce under the constraint of the so-called diminishing marginal product of labour. So adding an extra worker will reduce productivity because they assume the available capital that workers get to use is fixed in the short-run.

This assertion which does not stack up in the real world, yields the downward sloping marginal product of labour (the contribution of the last worker to production) relationship in the textbook model. Then profit maximising firms set the marginal product equal to the real wage to determine their employment decisions.

They do this because the marginal product is what the last worker produces (at the margin) and the real wage is what the worker costs in real terms to hire.

So when they have screwed the last bit of production out of the last worker hired and it equals the real wage, they have thus made “real gains” on all previous workers employed and cannot do any better – hence, they are said to have maximised profits.
Labour demand is thus inverse to the real wage. As the real wage rises, employment falls in this model because the marginal product falls with employment.

The simplest version is that labour supply in the mainstream model (and complex versions don’t add anything anyway) says that households equate the marginal disutility of work (the slope of the labour supply function) with the real wage (indicating the opportunity cost of leisure) to determine their utility maximising labour supply.

So in English, it is assumed that workers hate work and but like leisure (non-work). They will only go to work to get an income and the higher the real wage the more work they will supply because for each hour of labour supplied their prospective income is higher. Again, this conception is arbitrary and not consistent with countless empirical studies which show the total labour supply is more or less invariant to movements in the real wage.

Other more complex variations of the mainstream model depict labour supply functions with both non-zero real wage elasticities and, consistent with recent real business cycle analysis, sensitivity to the real interest rate. All ridiculous. Ignore them!

In the mainstream model, labour market clearing – that is when all firms who want to hire someone can find a worker to hire and all workers who want to work can find sufficient work – requires that the real wage equals the marginal product of labour. The real wage will change to ensure that this is maintained at all times thus providing the classical model with continuous full employment. So anything that prevents this from happening (government regulations) will create unemployment.

If a worker is “unemployed” then it must mean they desire a real wage that is excessive in relation to their productivity. The other way the mainstream characterise this is that the worker values leisure greater than income (work).

The equilibrium employment levels thus determine via the technological state of the economy (productivity function) the equilibrium (or full employment) level of aggregate supply of real output. So once all the labour markets are cleared the total level of output that is produced (determined by the productivity levels) will equal total output or GDP.

It was of particular significance for Keynes that the classical explanation for real output determination did not depend on the aggregate demand for it at all.

He argued that firms will not produce output that they do not think they will sell. So for him, total supply of GDP must be determined by aggregate demand (which he called effective demand – spending plans backed by a willingness to impart cash).

In the General Theory, Keynes questioned whether wage reductions could be readily achieved and was sceptical that, even if they could, employment would rise.

The adverse consequences for the effective demand for output were his principal concern.

So Keynes proposed the revolutionary idea (at the time) that employment was determined by effective monetary demand for output. Since there was no reason why the total demand for output would necessarily correspond to full employment, involuntary unemployment was likely.

Keynes revived Marx’s earlier works on effective demand (although he didn’t acknowledge that in his work – being anti-Marxist). What determined effective demand? There were two major elements: the consumption demand of households, and the investment demands of business.

So demand for aggregate output determined production levels which in turn determined total employment.

Keynes model reversed the classical causality in the macroeconomy. Demand determined output. Production levels then determined employment based on the current level of productivity. The labour market is then constrained by this level of employment demand. At the current money wage level, the level of unemployment (supply minus demand) is then determined. The firms will not expand employment unless the aggregate constraint is relaxed.

Keynes also argued that in a recession, the real wage might not fall because workers bargain for money or nominal wages, not real wages. The act of dropping money wages across the board would also reduce aggregate demand and prices would also fall. So there was no guarantee that real wages (the ratio of wages to prices) would therefore fall. They may rise or stay about the same.

Falling prices might, however, depress business profit expectations and so cut into demand for investment. This would actually reduce the demand for workers and prevent total employment from rising. The system interacts with itself, and an equilibrium of full employment cannot be achieved within the labour market.

Keynes also claimed that in a recession it should be clear that the problem is not that the real wage is too high, but rather that the prices are too low (as prices fall with lower production).

However, in Keynes’ analysis, attempting to cut real wages by cutting nominal wages would be resisted by the workers because they will not promote higher employment or output and also would imperil their ability to service their nominal contractual commitments (like mortgages). The argument is that workers will tolerate a fall in real wages brought about by prices rising faster than nominal wages because, within limits, they can still pay their nominal contractual obligations (by cutting back on other expenditure).

A more subtle point argued by Keynes is that wage cut resistance may be beneficial because of the distribution of income implications. If real wages fall, the share of real output claimed by the owners of capital (or non-labour fixed inputs) rises. Assuming such ownership is concentrated in a few hands, capitalists can be expected to have a higher propensity to save than the working class.

If so, aggregate saving from real output will increase and aggregate demand will fall further setting off a second round of oversupply of output and job losses.

It is also important to differentiate what happens if a firm lowers its wage level against what happens in the whole economy does the same. This relates to the so-called interdependence of demand and supply curves.

The mainstream model claims that the two sides of the market are independent so that a supply shift will not cause the demand side of the market to shift. So in this context, if a firm can lower its money wage rates it would not expect a major fall in the demand for its products because its workforce are a small proportion of total employment and their incomes are a small proportion of total demand.

If so, the firm can reduce its prices and may enjoy rising demand for its output and hence put more workers on. So the demand and supply of output are independent.

However there are solid reasons why firms will not want to behave like this. They get the reputation of being a capricious employer and will struggle to retain labour when the economy improves. Further, worker morale will fall and with it productivity. Other pathologies such as increased absenteeism etc would accompany this sort of firm behaviour.

But if the whole economy takes a wage cut, then while wage are a cost on the supply side they are an income on the demand side. So a cut in wages may reduce supply costs but also will reduce demand for output. In this case the aggregate demand and supply are interdependent and this violates the mainstream depiction.

This argument demonstrates one of the famous fallacies of composition in mainstream theory. That is, policies that might work at the micro (firm/sector) level will not generalise to work at the macroeconomic level.

There was much more to the Keynes versus the Classics debate but the general idea is as presented.
MMT integrates the insights of Keynes and others into a broader monetary framework. But the essential point is that mass unemployment is a macroeconomic phenomenon and trying to manipulate wage levels (relative to prices) will only change output and employment at the macroeconomic level if changes in demand are achieved as saving desires of the non-government sector respond.

It is highly unlikely for all the reasons noted that cutting real wages will reduce the non-government desire to save.

MMT tells us that the introduction of state money (the currency issued by the government) introduces the possibility of unemployment. There is no unemployment in non-monetary economies. As a background to this discussion you might like to read this blog – Functional finance and modern monetary theory .

MMT shows that taxation functions to promote offers from private individuals to government of goods and services in return for the necessary funds to extinguish the tax liabilities.

So taxation is a way that the government can elicit resources from the non-government sector because the latter have to get $s to pay their tax bills. Where else can they get the $s unless the government spends them on goods and services provided by the non-government sector?

A sovereign government is never revenue constrained and so taxation is not required to “finance” public spending. The mainstream economists conceive of taxation as providing revenue to the government which it requires in order to spend. In fact, the reverse is the truth.

Government spending provides revenue to the non-government sector which then allows them to extinguish their taxation liabilities. So the funds necessary to pay the tax liabilities are provided to the non-government sector by government spending.

It follows that the imposition of the taxation liability creates a demand for the government currency in the non-government sector which allows the government to pursue its economic and social policy program.

The non-government sector will seek to sell goods and services (including labour) to the government sector to get the currency (derived from the government spending) in order to extinguish its tax obligations to government as long as the tax regime is legally enforceable. Under these circumstances, the non-government sector will always accept government money because it is the means to get the $s necessary to pay the taxes due.

This insight allows us to see another dimension of taxation which is lost in mainstream economic analysis. Given that the non-government sector requires fiat currency to pay its taxation liabilities, in the first instance, the imposition of taxes (without a concomitant injection of spending) by design creates unemployment (people seeking paid work) in the non-government sector.

The unemployed or idle non-government resources can then be utilised through demand injections via government spending which amounts to a transfer of real goods and services from the non-government to the government sector.

In turn, this transfer facilitates the government’s socio-economics program. While real resources are transferred from the non-government sector in the form of goods and services that are purchased by government, the motivation to supply these resources is sourced back to the need to acquire fiat currency to extinguish the tax liabilities.

Further, while real resources are transferred, the taxation provides no additional financial capacity to the government of issue.
Conceptualising the relationship between the government and non-government sectors in this way makes it clear that it is government spending that provides the paid work which eliminates the unemployment created by the taxes.

So it is now possible to see why mass unemployment arises. It is the introduction of State Money (defined as government taxing and spending) into a non-monetary economy that raises the spectre of involuntary unemployment.

As a matter of accounting, for aggregate output to be sold, total spending must equal the total income generated in production (whether actual income generated in production is fully spent or not in each period).

Involuntary unemployment is idle labour offered for sale with no buyers at current prices (wages). Unemployment occurs when the private sector, in aggregate, desires to earn the monetary unit of account through the offer of labour but doesn’t desire to spend all it earns, other things equal.

As a result, involuntary inventory accumulation among sellers of goods and services translates into decreased output and employment.

In this situation, nominal (or real) wage cuts per se do not clear the labour market, unless those cuts somehow eliminate the private sector desire to net save, and thereby increase spending.

So we are now seeing that at a macroeconomic level, manipulating wage levels (or rates of growth) would not seem to be an effective strategy to solve mass unemployment.

MMT then concludes that mass unemployment occurs when net government spending is too low.

To recap: The purpose of State Money is to facilitate the movement of real goods and services from the non-government (largely private) sector to the government (public) domain.

Government achieves this transfer by first levying a tax, which creates a notional demand for its currency of issue.

To obtain funds needed to pay taxes and net save, non-government agents offer real goods and services for sale in exchange for the needed units of the currency. This includes, of-course, the offer of labour by the unemployed.

The obvious conclusion is that unemployment occurs when net government spending is too low to accommodate the need to pay taxes and the desire to net save.

This analysis also sets the limits on government spending. It is clear that government spending has to be sufficient to allow taxes to be paid. In addition, net government spending is required to meet the private desire to save (accumulate net financial assets).

It is also clear that if the Government doesn’t spend enough to cover taxes and the non-government sector’s desire to save the manifestation of this deficiency will be unemployment.

Keynesians have used the term demand-deficient unemployment. In MMT, the basis of this deficiency is at all times inadequate net government spending, given the private spending (saving) decisions in force at any particular time.

Shift in private spending certainly lead to job losses but the persistent of these job losses is all down to inadequate net government spending.

But in terms of the question – after all that – it is clear that excessive real wages could impinge on the rate of profit that the capitalists desired and if they translate that into a cut back in investment then aggregate demand might fall. Note: this explanation has nothing to do with the standard mainstream textbook explanation. It is totally consistent with MMT and the Keynesian story – output and employment is determined by aggregate demand and anything that impacts adversely on the latter will undermine employment.

The following blogs may be of further interest to you:

• Functional finance and modern monetary theory
• What causes mass unemployment?
• Modern monetary theory in an open economy
• Deficit spending 101 – Part 1
• Deficit spending 101 – Part 2
• Deficit spending 101 – Part 3

Question 3:

For workers to get a rising share of national income their nominal wages have to grow faster than inflation.

The answer is False.

The wage share in nominal GDP is expressed as the total wage bill as a percentage of nominal GDP. Economists differentiate between nominal GDP ($GDP), which is total output produced at market prices and real GDP (GDP), which is the actual physical equivalent of the nominal GDP. We will come back to that distinction soon.

To compute the wage share we need to consider total labour costs in production and the flow of production ($GDP) each period.

Employment (L) is a stock and is measured in persons (averaged over some period like a month or a quarter or a year.

The wage bill is a flow and is the product of total employment (L) and the average wage (w) prevailing at any point in time. Stocks (L) become flows if it is multiplied by a flow variable (W). So the wage bill is the total labour costs in production per period.

So the wage bill = W.L

The wage share is just the total labour costs expressed as a proportion of $GDP – (W.L)/$GDP in nominal terms, usually expressed as a percentage. We can actually break this down further.

Labour productivity (LP) is the units of real GDP per person employed per period. Using the symbols already defined this can be written as:

LP = GDP/L

so it tells us what real output (GDP) each labour unit that is added to production produces on average.

We can also define another term that is regularly used in the media – the real wage – which is the purchasing power equivalent on the nominal wage that workers get paid each period. To compute the real wage we need to consider two variables: (a) the nominal wage (W) and the aggregate price level (P).

We might consider the aggregate price level to be measured by the consumer price index (CPI) although there are huge debates about that. But in a sense, this macroeconomic price level doesn’t exist but represents some abstract measure of the general movement in all prices in the economy.

Macroeconomics is hard to learn because it involves these abstract variables that are never observed – like the price level, like “the interest rate” etc. They are just stylisations of the general tendency of all the different prices and interest rates.

Now the nominal wage (W) – that is paid by employers to workers is determined in the labour market – by the contract of employment between the worker and the employer. The price level (P) is determined in the goods market – by the interaction of total supply of output and aggregate demand for that output although there are complex models of firm price setting that use cost-plus mark-up formulas with demand just determining volume sold. We shouldn’t get into those debates here.

The inflation rate is just the continuous growth in the price level (P). A once-off adjustment in the price level is not considered by economists to constitute inflation.

So the real wage (w) tells us what volume of real goods and services the nominal wage (W) will be able to command and is obviously influenced by the level of W and the price level. For a given W, the lower is P the greater the purchasing power of the nominal wage and so the higher is the real wage (w).

We write the real wage (w) as W/P. So if W = 10 and P = 1, then the real wage (w) = 10 meaning that the current wage will buy 10 units of real output. If P rose to 2 then w = 5, meaning the real wage was now cut by one-half.

So the proposition in the question – that nominal wages grow faster than inflation – tells us that the real wage is rising.

Nominal GDP ($GDP) can be written as P.GDP, where the P values the real physical output.

Now if you put of these concepts together you get an interesting framework. To help you follow the logic here are the terms developed and be careful not to confuse $GDP (nominal) with GDP (real):

• Wage share = (W.L)/$GDP
• Nominal GDP: $GDP = P.GDP
• Labour productivity: LP = GDP/L
• Real wage: w = W/P

By substituting the expression for Nominal GDP into the wage share measure we get:

Wage share = (W.L)/P.GDP

In this area of economics, we often look for alternative way to write this expression – it maintains the equivalence (that is, obeys all the rules of algebra) but presents the expression (in this case the wage share) in a different “view”.

So we can write as an equivalent:

Wage share – (W/P).(L/GDP)

Now if you note that (L/GDP) is the inverse (reciprocal) of the labour productivity term (GDP/L). We can use another rule of algebra (reversing the invert and multiply rule) to rewrite this expression again in a more interpretable fashion.

So an equivalent but more convenient measure of the wage share is:

Wage share = (W/P)/(GDP/L) – that is, the real wage (W/P) divided by labour productivity (GDP/L).

I won’t show this but I could also express this in growth terms such that if the growth in the real wage equals labour productivity growth the wage share is constant. The algebra is simple but we have done enough of that already.

That journey might have seemed difficult to non-economists (or those not well-versed in algebra) but it produces a very easy to understand formula for the wage share.

Two other points to note. The wage share is also equivalent to the real unit labour cost (RULC) measures that Treasuries and central banks use to describe trends in costs within the economy. Please read my blog – Saturday Quiz – May 15, 2010 – answers and discussion – for more discussion on this point.

Now it becomes obvious that if the nominal wage (W) grows faster than the price level (P) then the real wage is growing. But that doesn’t automatically lead to a growing wage share. So the blanket proposition stated in the question is false.

If the real wage is growing at the same rate as labour productivity, then both terms in the wage share ratio are equal and so the wage share is constant.

If the real wage is growing but labour productivity is growing faster, then the wage share will fall.

Only if the real wage is growing faster than labour productivity , will the wage share rise.

The wage share was constant for a long time during the Post Second World period and this constancy was so marked that Kaldor (the Cambridge economist) termed it one of the great “stylised” facts. So real wages grew in line with productivity growth which was the source of increasing living standards for workers.

The productivity growth provided the “room” in the distribution system for workers to enjoy a greater command over real production and thus higher living standards without threatening inflation.

Since the mid-1980s, the neo-liberal assault on workers’ rights (trade union attacks; deregulation; privatisation; persistently high unemployment) has seen this nexus between real wages and labour productivity growth broken. So while real wages have been stagnant or growing modestly, this growth has been dwarfed by labour productivity growth.

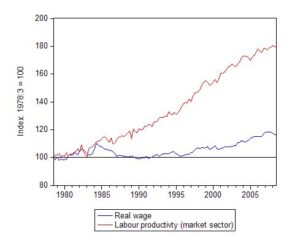
In this blog – The origins of the economic crisis – I provided these graphs. First, the movement real wages and labour productivity since 1979. Both series are indexed to 100 as at the September quarter 1978. So by September 2008, the real wage index had climbed to 116.7 (that is, around 15 per cent growth in just over 12 years) but the labour productivity index was 179.1.

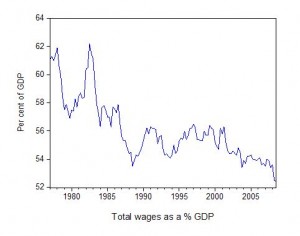
This suggests from our discussion that the wage share should have fallen. That is what the next graph depicts – it shows how far the wage share has fallen in

Australia over the last two decades. The trend has been common across the globe during the neo-liberal years and is one of the pre-conditions that explain our current economic crisis.

Question 4:

Modern Monetary Theory implies that higher levels of taxation are not necessary for the government to spend more in real terms.

The answer is False.

Modern Monetary Theory (MMT) shows that taxpayers do fund anything and sovereign governments are never revenue-constrained because they are the monopoly issuers of the currency in use. Therefore, the government can spend whatever it likes irrespective of the level of taxation. Therefore if you didn’t really think beyond that you would conclude that the answer was True. And you would be wrong.

That logic while correct for the most part ignores the underlying role of taxation.

In a fiat monetary system the currency has no intrinsic worth. Further the government has no intrinsic financial constraint. Once we realise that government spending is not revenue-constrained then we have to analyse the functions of taxation in a different light. The starting point of this new understanding is that taxation functions to promote offers from private individuals to government of goods and services in return for the necessary funds to extinguish the tax liabilities.

In this way, it is clear that the imposition of taxes creates unemployment (people seeking paid work) in the non-government sector and allows a transfer of real goods and services from the non-government to the government sector, which in turn, facilitates the government’s economic and social program.

The crucial point is that the funds necessary to pay the tax liabilities are provided to the non-government sector by government spending. Accordingly, government spending provides the paid work which eliminates the unemployment created by the taxes.

This train of logic also explains why mass unemployment arises. It is the introduction of State Money (government taxing and spending) into a non-monetary economics that raises the spectre of involuntary unemployment. For aggregate output to be sold, total spending must equal total income (whether actual income generated in production is fully spent or not each period). Involuntary unemployment is idle labour offered for sale with no buyers at current prices (wages).

Unemployment occurs when the private sector, in aggregate, desires to earn the monetary unit of account, but doesn’t desire to spend all it earns, other things equal. As a result, involuntary inventory accumulation among sellers of goods and services translates into decreased output and employment. In this situation, nominal (or real) wage cuts per se do not clear the labour market, unless those cuts somehow eliminate the private sector desire to net save, and thereby increase spending.

The purpose of State Money is for the government to move real resources from private to public domain. It does so by first levying a tax, which creates a notional demand for its currency of issue. To obtain funds needed to pay taxes and net save, non-government agents offer real goods and services for sale in exchange for the needed units of the currency. This includes, of-course, the offer of labour by the unemployed. The obvious conclusion is that unemployment occurs when net government spending is too low to accommodate the need to pay taxes and the desire to net save.

This analysis also sets the limits on government spending. It is clear that government spending has to be sufficient to allow taxes to be paid. In addition, net government spending is required to meet the private desire to save (accumulate net financial assets). From the previous paragraph it is also clear that if the Government doesn’t spend enough to cover taxes and desire to save the manifestation of this deficiency will be unemployment.

Keynesians have used the term demand-deficient unemployment. In our conception, the basis of this deficiency is at all times inadequate net government spending, given the private spending decisions in force at any particular time.

Accordingly, the concept of fiscal sustainability does not entertain notions that the continuous deficits required to finance non-government net saving desires in the currency of issue will ultimately require high taxes. Taxes in the future might be higher or lower or unchanged. These movements have nothing to do with “funding” government spending.

To understand how taxes are used to attenuate demand please read this blog – Functional finance and modern monetary theory.

So to make the point clear – the taxes do not fund the spending. But they do free up space for the spending to occur in a non-inflationary environment.

You might say that the answer is only false when the economy is at full employment where there are no free resources. In that context, taxation has to take those resources off the non-government sector in order for the government to spend more. That is a true statement.

But it doesn’t negate the overall falsity of the main proposition.

Further, you might say that governments can spend whenever they like. That is also true but if it just kept spending the growth in nominal demand would outstrip real capacity and inflation would certainly result. So in that regard, this would not be a sensible strategy and is excluded as a reasonable proposition. In addition, that angle is covered by the “real terms” component of the question.

So on balance the sensible answer is false.

The following blogs may be of further interest to you:

• A modern monetary theory lullaby
• Functional finance and modern monetary theory
• Deficit spending 101 – Part 1
• Deficit spending 101 – Part 2
• Deficit spending 101 – Part 3

Premium Question 5:

After a deep recession, governments wanting to reduce their unemployment rates should use expansionary fiscal policy to get real GDP growth back on trend.

The answer is Maybe.

To see why, we might usefully construct a scenario that will explicate the options available to a government:

• Trend real GDP growth rate is 3 per cent annum.
• Labour productivity growth (that is, growth in real output per person employed) is growing at 2 per cent per annum. So as this grows less employment in required per unit of output.
• The labour force is growing by 1.5 per cent per annum. Growth in the labour force adds to the employment that has to be generated for unemployment to stay constant (or fall).
• The average working week is constant in hours. So firms are not making hours adjustments up or down with their existing workforce. Hours adjustments alter the relationship between real GDP growth and persons employed.

We can use this scenario to explore the different outcomes.

The trend rate of real GDP growth doesn’t relate to the labour market in any direct way. The late Arthur Okun is famous (among other things) for estimating the relationship that links the percentage deviation in real GDP growth from potential to the percentage change in the unemployment rate – the so-called Okun’s Law.

The algebra underlying this law can be manipulated to estimate the evolution of the unemployment rate based on real output forecasts.

From Okun, we can relate the major output and labour-force aggregates to form expectations about changes in the aggregate unemployment rate based on output growth rates. A series of accounting identities underpins Okun’s Law and helps us, in part, to understand why unemployment rates have risen.

Take the following output accounting statement:

(1) Y = LP*(1-UR)LH

where Y is real GDP, LP is labour productivity in persons (that is, real output per unit of labour), H is the average number of hours worked per period, UR is the aggregate unemployment rate, and L is the labour-force. So (1-UR) is the employment rate, by definition.

Equation (1) just tells us the obvious – that total output produced in a period is equal to total labour input [(1-UR)LH] times the amount of output each unit of labour input produces (LP).

Using some simple calculus you can convert Equation (1) into an approximate dynamic equation expressing percentage growth rates, which in turn, provides a simple benchmark to estimate, for given labour-force and labour productivity growth rates, the increase in output required to achieve a desired unemployment rate.

Accordingly, with small letters indicating percentage growth rates and assuming that the average number of hours worked per period is more or less constant, we get:

(2) y = lp + (1 – ur) + lf

Re-arranging Equation (2) to express it in a way that allows us to achieve our aim (re-arranging just means taking and adding things to both sides of the equation):

(3) ur = 1 + lp + lf – y

Equation (3) provides the approximate rule of thumb – if the unemployment rate is to remain constant, the rate of real output growth must equal the rate of growth in the labour-force plus the growth rate in labour productivity.

It is an approximate relationship because cyclical movements in labour productivity (changes in hoarding) and the labour-force participation rates can modify the relationships in the short-run. But it provides reasonable estimates of what happens when real output changes.

The sum of labour force and productivity growth rates is referred to as the required real GDP growth rate – required to keep the unemployment rate constant.

Remember that labour productivity growth (real GDP per person employed) reduces the need for labour for a given real GDP growth rate while labour force growth adds workers that have to be accommodated for by the real GDP growth (for a given productivity growth rate).

So in the example, the required real GDP growth rate is 3.5 per cent per annum and if policy only aspires to keep real GDP growth at its trend growth rate of 3 per cent annum, then the output gap that emerges is 0.5 per cent per annum.

The unemployment rate will rise by this much (give or take) and reflects the fact that real output growth is not strong enough to both absorb the new entrants into the labour market and offset the employment losses arising from labour productivity growth.

The following blog may be of further interest to you:

• What do the IMF growth projections mean?