The Weekend Quiz – January 5-6 – answers and discussion

January 5, 2019
bill
Saturday quiz
0 Comments

Here are the answers with discussion for this Weekend’s Quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of Modern Monetary Theory (MMT) and its application to macroeconomic thinking. Comments as usual welcome, especially if I have made an error.

Question 1:

If inflation rate remained equal to the nominal interest rate, then the government primary deficit could double (from say 2 to 4 per cent of GDP) without pushing up public debt as a proportion of GDP.

The answer is True.

Again, this question requires a careful reading and a careful association of concepts to make sure they are commensurate.

There are two concepts that are central to the question: (a) a rising fiscal deficit – which is a flow and not scaled by GDP in this case; and (b) a rising public debt ratio which by construction (as a ratio) is scaled by GDP.

So the two concepts are not commensurate although they are related in some way.

A rising fiscal deficit does not necessary lead to a rising public debt ratio. You might like to refresh your understanding of these concepts by reading this blog – Saturday Quiz – March 6, 2010 – answers and discussion.

While the mainstream macroeconomics thinks that a sovereign government is revenue-constrained and is subject to the ‘government budget constraint’, MMT places no particular importance in the public debt to GDP ratio for a sovereign government, given that insolvency is not an issue.

The mainstream framework for analysing the so-called ‘financing’ choices faced by a government (taxation, debt-issuance, money creation) is written as:

Which you can read in English as saying that fiscal deficit (BD) equals Government spending (G_t) plus Government interest payments (rB_t-1 minus Tax receipts (T_t) which must equal (be ‘financed’ by) a change in new bonds issued (δB_t) and/or a change in high powered money (δH_t).

The triangle sign (delta) is just shorthand for the change in a variable. The subscript t is this period, whereas t-1 is last period.

Remember, this is merely an accounting statement

In a stock-flow consistent macroeconomics, this statement will always hold. That is, it has to be true if all the transactions between the government and non-government sector have been corrected added and subtracted.

So from the perspective of Modern Monetary Theory (MMT), the previous equation is just an ex post accounting identity that has to be true by definition and has not real economic importance.

For the mainstream economist, the equation represents an ex ante (before the fact) financial constraint that the government is bound by.

The difference between these two conceptions is very significant and the second (mainstream) interpretation cannot be correct if governments issue fiat currency (unless they place voluntary constraints on themselves to act as if it is).

The accounting relationship can be manipulated to provide an expression linking deficits and changes in the public debt ratio.

The following equation expresses the relationships above as proportions of GDP:

So the change in the debt ratio is the sum of two terms on the right-hand side: (a) the difference between the real interest rate (r) and the GDP growth rate (g) times the initial debt ratio; and (b) the ratio of the primary deficit (G-T) to GDP.

A primary fiscal balance is the difference between government spending (excluding interest rate servicing) and taxation revenue.

The real interest rate is the difference between the nominal interest rate and the inflation rate. If inflation is maintained at a rate equal to the interest rate then the real interest rate is constant.

A growing economy can absorb more debt and keep the debt ratio constant or falling.

From the formula above, if the primary fiscal balance is zero, public debt increases at a rate r but the public debt ratio increases at r – g.

So if r = 0, and g = 2, the primary deficit ratio could equal 2 per cent (of GDP) and the public debt ratio would be unchanged. Doubling the primary deficit to 4 per cent would require g to rise to 4 for the public debt ratio to remain unchanged. That is entirely possible.

So a nation running a primary deficit can obviously reduce its public debt ratio over time or hold them constant if growth is stimulated. Further, you can see that even with a rising primary deficit, if output growth (g) is sufficiently greater than the real interest rate (r) then the debt ratio can fall from its value last period.

Furthermore, depending on contributions from the external sector, a nation running a deficit will more likely create the conditions for a reduction in the public debt ratio than a nation that introduces an austerity plan aimed at running primary surpluses.

Clearly, the real growth rate has limits and that would limit the ability of a government (that voluntarily issues debt) to hold the debt ratio constant while expanding its primary fiscal deficit as a proportion of GDP.

The following blog may be of further interest to you:

Saturday Quiz – March 6, 2010 – answers and discussion

Question 2:

If the share of wages in national income falls then the workers’ material standard of living also falls.

The answer is False.

The wage share in nominal GDP is expressed as the total wage bill as a percentage of nominal GDP. Economists differentiate between nominal GDP ($GDP), which is total output produced at market prices and real GDP (GDP), which is the actual physical equivalent of the nominal GDP. We will come back to that distinction soon.

To compute the wage share we need to consider total labour costs in production and the flow of production ($GDP) each period.

Employment (L) is a stock and is measured in persons (averaged over some period like a month or a quarter or a year.

The wage bill is a flow and is the product of total employment (L) and the average wage (w) prevailing at any point in time. Stocks (L) become flows if it is multiplied by a flow variable (W). So the wage bill is the total labour costs in production per period.

So the wage bill = W.L

The wage share is just the total labour costs expressed as a proportion of $GDP – (W.L)/$GDP in nominal terms, usually expressed as a percentage. We can actually break this down further.

Labour productivity (LP) is the units of real GDP per person employed per period. Using the symbols already defined this can be written as:

LP = GDP/L

so it tells us what real output (GDP) each labour unit that is added to production produces on average.

We can also define another term that is regularly used in the media – the real wage – which is the purchasing power equivalent on the nominal wage that workers get paid each period. To compute the real wage we need to consider two variables: (a) the nominal wage (W) and the aggregate price level (P).

We might consider the aggregate price level to be measured by the consumer price index (CPI) although there are huge debates about that. But in a sense, this macroeconomic price level doesn’t exist but represents some abstract measure of the general movement in all prices in the economy.

The real wage (w) tells us what volume of real goods and services the nominal wage (W) will be able to command and is obviously influenced by the level of W and the price level. For a given W, the lower is P the greater the purchasing power of the nominal wage and so the higher is the real wage (w).

We write the real wage (w) as W/P. So if W = 10 and P = 1, then the real wage (w) = 10 meaning that the current wage will buy 10 units of real output. If P rose to 2 then w = 5, meaning the real wage was now cut by one-half.

So the proposition in the question – that a declining wage share does not mean the real standard of living for workers is falling – true.

Irrespective of what happens to the wage share, as long as the real wage is rising, material standards of living will be rising (other things equal). That is, a declining wage share per se doesn’t denote a decline in workers’ living standards.

What it tells us is that a rising proportion of national income is going to profits (non-wages). But that rising proportion could be in relation to an overall expanding pie.

A declining wage share is consistent with growth in the real wage which is slower than the growth in labour productivity. If the real wage is growing but labour productivity is growing faster, then the wage share will fall.

A declining wage share driven by a real wage falling (and labour productivity at least not falling by as much) would signify a decline in living standards but that is because the real wage is falling.

The following blogs may be of further interest to you:

Question 3:

Assume that inflation is stable, there is excess productive capacity, and the central bank maintains its current interest rate target. If on average the government collects an income tax of 20 cents in the dollar, then total tax revenue will rise by 0.20 times $x if government spending increases (once and for all) by $x dollars and private investment and exports remain unchanged.

The answer is False.

This question relates to the concept of a spending multiplier and the relationship between spending injections and spending leakages.

We have made the question easy by assuming that only government spending changes (exogenously) in period one and then remains unchanged after that – that is, a once and for all increase.

Aggregate demand drives output which then generates incomes (via payments to the productive inputs). Accordingly, what is spent will generate income in that period which is available for use. The uses are further consumption, paying taxes and/or buying imports.

We consider imports as a separate category (even though they reflect consumption, investment and government spending decisions) because they constitute spending which does not recycle back into the production process. They are thus considered to be ‘leakages’ from the expenditure system.

So if for every dollar produced and paid out as income, if the economy imports around 20 cents in the dollar, then only 80 cents is available within the system for spending in subsequent periods excluding taxation considerations.

However there are two other ‘leakages’ which arise from domestic sources – saving and taxation. Take taxation first. When income is produced, the households end up with less than they are paid out in gross terms because the government levies a tax. So the income concept available for subsequent spending is called disposable income (Yd).

In the example we assumed an average tax rate of 20 cents in the dollar is levied (which is equivalent to a proportional tax rate of 0.20). So if $100 of new income is generated, $20 goes to taxation and Yd is $80 (what is left). So taxation (T) is a ‘leakage’ from the expenditure system in the same way as imports are.

You were induced to think along those lines.

But the answer relies us to understand what is the new income generated from the once-off injection of %x dollars?

The concept of the spending multiplier tells us that the final change in income will exceed the initial injection (in the question $x dollars).

Finally consider saving. Consumers make decisions to spend a proportion of their disposable income. The amount of each dollar they spent at the margin (that is, how much of every extra dollar to they consume) is called the marginal propensity to consume. If that is 0.80 then they spent 80 cents in every dollar of disposable income.

So if total disposable income is $80 (after taxation of 20 cents in the dollar is collected) then consumption (C) will be 0.80 times $80 which is $64 and saving will be the residual – $26. Saving (S) is also a “leakage” from the expenditure system.

It is easy to see that for every $100 produced, the income that is generated and distributed results in $64 in consumption and $36 in leakages which do not cycle back into spending.

For income to remain at the higher level (after the extra $100 is created)in the next period the $36 has to be made up by what economists call ‘injections’ which in these sorts of models comprise the sum of investment (I), government spending (G) and exports (X). The injections are seen as coming from “outside” the output-income generating process (they are called exogenous or autonomous expenditure variables).

For GDP to be stable injections have to equal leakages (this can be converted into growth terms to the same effect). The national accounting statements that we have discussed previous such that the government deficit (surplus) equals $-for-$ the non-government surplus (deficit) and those that decompose the non-government sector in the external and private domestic sectors is derived from these relationships.

So imagine there is a certain level of income being produced – its value is immaterial. Imagine that the central bank sees no inflation risk and so interest rates are stable as are exchange rates (these simplifications are to to eliminate unnecessary complexity).

The question then is: what would happen if government increased spending by, say, $100? This is the terrain of the multiplier. If aggregate demand increases drive higher output and income increases then the question is by how much?

The spending multiplier is defined as the change in real income that results from a dollar change in exogenous aggregate demand (so one of G, I or X). We could complicate this by having autonomous consumption as well but the principle is not altered.

So the starting point is to define the consumption relationship. The most simple is a proportional relationship to disposable income (Yd). So we might write it as C = c*Yd – where little c is the marginal propensity to consume (MPC) or the fraction of every dollar of disposable income consumed. We will use c = 0.8.

The * sign denotes multiplication. You can do this example in an spreadsheet if you like.

Our tax relationship is already defined above – so T = tY. The little t is the marginal tax rate which in this case is the proportional rate (assume it is 0.2). Note here taxes are taken out of total income (Y) which then defines disposable income.

So Yd = (1-t) times Y or Yd = (1-0.2)*Y = 0.8*Y

If imports (M) are 20 per cent of total income (Y) then the relationship is M = m*Y where little m is the marginal propensity to import or the economy will increase imports by 20 cents for every real GDP dollar produced.

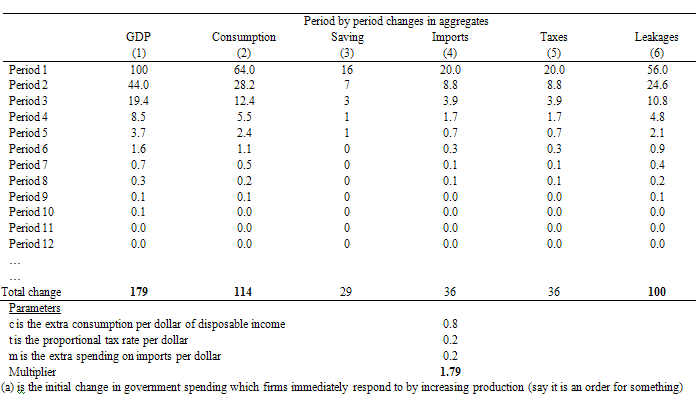
If you understand all that then the explanation of the multiplier follows logically. Imagine that government spending went up by $100 and the change in real national income is $179. Then the multiplier is the ratio (denoted k) of the Change in Total Income to the Change in government spending.

Thus k = $179/$100 = 1.79.

This says that for every dollar the government spends total real GDP will rise by $1.79 after taking into account the leakages from taxation, saving and imports.

When we conduct this thought experiment we are assuming the other autonomous expenditure components (I and X) are unchanged.

But the important point is to understand why the process generates a multiplier value of 1.79.

Here is a spreadsheet table I produced as a basis of the explanation. You might want to click it and then print it off if you are having trouble following the period by period flows.

So at the start of Period 1, the government increases spending by $100. The Table then traces out the changes that occur in the macroeconomic aggregates that follow this increase in spending (and “injection” of $100). The total change in real GDP (Column 1) will then tell us the multiplier value (although there is a simple formula that can compute it). The parameters which drive the individual flows are shown at the bottom of the table.

Note I have left out the full period adjustment – only showing up to Period 12. After that the adjustments are tiny until they peter out to zero.

Firms initially react to the $100 order from government at the beginning of the process of change. They increase output (assuming no change in inventories) and generate an extra $100 in income as a consequence which is the 100 change in GDP in Column [1].

The government taxes this income increase at 20 cents in the dollar (t = 0.20) and so disposable income only rises by $80 (Column 5).

There is a saying that one person’s income is another person’s expenditure and so the more the latter spends the more the former will receive and spend in turn – repeating the process.

Households spend 80 cents of every disposable dollar they receive which means that consumption rises by $64 in response to the rise in production/income. Households also save $16 of disposable income as a residual.

Imports also rise by $20 given that every dollar of GDP leads to a 20 cents increase imports (by assumption here) and this spending is lost from the spending stream in the next period.

So the initial rise in government spending has induced new consumption spending of $64. The workers who earned that income spend it and the production system responds. But remember $20 was lost from the spending stream via imports so the second period spending increase is $44. Firms react and generate and extra $44 to meet the increase in aggregate demand.

And so the process continues with each period seeing a smaller and smaller induced spending effect (via consumption) because the leakages are draining the spending that gets recycled into increased production.

Eventually the process stops and income reaches its new “equilibrium” level in response to the step-increase of $100 in government spending. Note I haven’t show the total process in the Table and the final totals are the actual final totals.

If you check the total change in leakages (S + T + M) in Column (6) you see they equal $100 which matches the initial injection of government spending. The rule is that the multiplier process ends when the sum of the change in leakages matches the initial injection which started the process off.

You can also see that the initial injection of government spending ($100) stimulates an eventual rise in GDP of $179 (hence the multiplier of 1.79) and consumption has risen by 114, Saving by 29 and Imports by 36.

The total tax take is thus $36 after the multiplier process is exhausted. For those who are familiar with algebra, the total change in teax revenue is equal to 0.2*1.79*$X, which in English says equals the tax rate times the multiplied initial change in aggregate demand.

So while the overall rise in nominal income is greater than the initial injection as a result of the multiplier that income increase produces leakages which sum to that exogenous spending impulse. At that point, the income expansion ceases.

The following blogs may be of further interest to you:

That is enough for today!