Here are the answers with discussion for this Weekend’s Quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of Modern…
The Weekend Quiz – May 21-22, 2022 – answers and discussion
Here are the answers with discussion for this Weekend’s Quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of Modern Monetary Theory (MMT) and its application to macroeconomic thinking. Comments as usual welcome, especially if I have made an error.
Question 1:
Central bankers are talking about ending their quantitative easing programs as an anti-inflationary strategy while governments are withdrawing of fiscal stimulus programs. Both policy shifts will destroy net financial assets in the non-government sector.
The answer is False.
Quantitative easing involves the central bank buying assets from the private sector – government bonds and high quality corporate debt.
So what the central bank is doing is swapping financial assets with the banks – they sell their financial assets and receive back extra reserves in return.
So the central bank is buying one type of financial asset (private holdings of bonds, company paper) and exchanging it for another (reserve balances at the central bank).
The net financial assets in the private sector are in fact unchanged although the portfolio composition of those assets is altered (maturity substitution) which changes yields and returns.
In terms of changing portfolio compositions, quantitative easing increases central bank demand for ‘long maturity’ assets held in the private sector which reduces interest rates at the longer end of the yield curve.
A reversal of the policy could take several forms.
(a) Refraining from additional purchases and allowing the government to pay out the debt – no impact on net financial assets in the non-government sector.
(b) Selling the bonds back to the private market, thus swapping bonds for reserves (the reverse of the original bond purchase).
Only the fiscal shift will impact on the net financial assets in the non-government sector.
The following blog posts may be of further interest to you:
- Money multiplier and other myths
- Islands in the sun
- Operation twist – then and now
- Quantitative easing 101
- Building bank reserves will not expand credit
- Building bank reserves is not inflationary
- Deficit spending 101 – Part 1
- Deficit spending 101 – Part 2
- Deficit spending 101 – Part 3
Question 2:
Australian workers found out officially this week that the real wage fell 2.7 per cent in the last 12 months. Given the growth in wages (the money you get paid) is lagging behind inflation while labour productivity is growing, then we can conclude that the profit share in GDP must be increasing.
The answer is True.
The wage share in nominal GDP is expressed as the total wage bill as a percentage of nominal GDP. Economists differentiate between nominal GDP ($GDP), which is total output produced at market prices and real GDP (GDP), which is the actual physical equivalent of the nominal GDP. We will come back to that distinction soon.
To compute the wage share we need to consider total labour costs in production and the flow of production ($GDP) each period.
Employment (L) is a stock and is measured in persons (averaged over some period like a month or a quarter or a year.
The wage bill is a flow and is the product of total employment (L) and the average wage (w) prevailing at any point in time. Stocks (L) become flows if it is multiplied by a flow variable (W). So the wage bill is the total labour costs in production per period.
So the wage bill = W.L
The wage share is just the total labour costs expressed as a proportion of $GDP – (W.L)/$GDP in nominal terms, usually expressed as a percentage. We can actually break this down further.
Labour productivity (LP) is the units of real GDP per person employed per period. Using the symbols already defined this can be written as:
LP = GDP/L
so it tells us what real output (GDP) each labour unit that is added to production produces on average.
We can also define another term that is regularly used in the media – the real wage – which is the purchasing power equivalent on the nominal wage that workers get paid each period. To compute the real wage we need to consider two variables: (a) the nominal wage (W) and the aggregate price level (P).
We might consider the aggregate price level to be measured by the consumer price index (CPI) although there are huge debates about that. But in a sense, this macroeconomic price level doesn’t exist but represents some abstract measure of the general movement in all prices in the economy.
Macroeconomics is hard to learn because it involves these abstract variables that are never observed – like the price level, like “the interest rate” etc. They are just stylisations of the general tendency of all the different prices and interest rates.
Now the nominal wage (W) – that is paid by employers to workers is determined in the labour market – by the contract of employment between the worker and the employer. The price level (P) is determined in the goods market – by the interaction of total supply of output and aggregate demand for that output although there are complex models of firm price setting that use cost-plus mark-up formulas with demand just determining volume sold. We shouldn’t get into those debates here.
The inflation rate is just the continuous growth in the price level (P). A once-off adjustment in the price level is not considered by economists to constitute inflation.
So the real wage (w) tells us what volume of real goods and services the nominal wage (W) will be able to command and is obviously influenced by the level of W and the price level. For a given W, the lower is P the greater the purchasing power of the nominal wage and so the higher is the real wage (w).
We write the real wage (w) as W/P. So if W = 10 and P = 1, then the real wage (w) = 10 meaning that the current wage will buy 10 units of real output. If P rose to 2 then w = 5, meaning the real wage was now cut by one-half.
Nominal GDP ($GDP) can be written as P.GDP, where the P values the real physical output.
Now if you put of these concepts together you get an interesting framework. To help you follow the logic here are the terms developed and be careful not to confuse $GDP (nominal) with GDP (real):
- Wage share = (W.L)/$GDP
- Nominal GDP: $GDP = P.GDP
- Labour productivity: LP = GDP/L
- Real wage: w = W/P
By substituting the expression for Nominal GDP into the wage share measure we get:
Wage share = (W.L)/P.GDP
In this area of economics, we often look for alternative way to write this expression – it maintains the equivalence (that is, obeys all the rules of algebra) but presents the expression (in this case the wage share) in a different “view”.
So we can write as an equivalent:
Wage share – (W/P).(L/GDP)
Now if you note that (L/GDP) is the inverse (reciprocal) of the labour productivity term (GDP/L). We can use another rule of algebra (reversing the invert and multiply rule) to rewrite this expression again in a more interpretable fashion.
So an equivalent but more convenient measure of the wage share is:
Wage share = (W/P)/(GDP/L) – that is, the real wage (W/P) divided by labour productivity (GDP/L).
I won’t show this but I could also express this in growth terms such that if the growth in the real wage equals labour productivity growth the wage share is constant. The algebra is simple but we have done enough of that already.
That journey might have seemed difficult to non-economists (or those not well-versed in algebra) but it produces a very easy to understand formula for the wage share.
Two other points to note. The wage share is also equivalent to the real unit labour cost (RULC) measures that Treasuries and central banks use to describe trends in costs within the economy. Please read my blog post – Saturday Quiz – May 15, 2010 – answers and discussion – for more discussion on this point.
Now it becomes obvious that if the nominal wage (W) and the price level (P) are growing at the pace the real wage is constant. And if the real wage is growing at the same rate as labour productivity, then both terms in the wage share ratio are equal and so the wage share is constant.
The wage share was constant for a long time during the Post Second World period and this constancy was so marked that Kaldor (the Cambridge economist) termed it one of the great “stylised” facts. So real wages grew in line with productivity growth which was the source of increasing living standards for workers.
The productivity growth provided the “room” in the distribution system for workers to enjoy a greater command over real production and thus higher living standards without threatening inflation.
Since the mid-1980s, the neo-liberal assault on workers’ rights (trade union attacks; deregulation; privatisation; persistently high unemployment) has seen this nexus between real wages and labour productivity growth broken. So while real wages have been stagnant or growing modestly, this growth has been dwarfed by labour productivity growth.
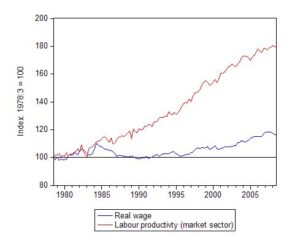
In this blog – The origins of the economic crisis – I provided these graphs. First, the movement real wages and labour productivity since 1979. Both series are indexed to 100 as at the September quarter 1978. So by September 2008, the real wage index had climbed to 116.7 (that is, around 15 per cent growth in just over 12 years) but the labour productivity index was 179.1.
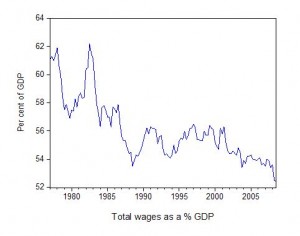
This suggests from our discussion that the wage share should have fallen. That is what the next graph depicts – it shows how far the wage share has fallen in Australia over the last two decades. The trend has been common across the globe during the neo-liberal years and is one of the pre-conditions that explain our current economic crisis.
The following blog posts may be of further interest to you:
Question 3
3. The government is attempting to reduce the fiscal deficit. Private market orientated advisors tell them to cut government spending, whereas, the more civic-minded advisors argue that it is better to increase taxes in an equitable way than to engage in harsh spending cuts. Which policy option – a tax rate increase that will increase tax revenue at the current level of national income by $x or a spending cut of $x – will have the greater initial impact on aggregate demand?
(a) Tax increase
(b) Spending cut
(c) Both will be equivalent
(d) There is not enough information to answer this question
The answer is Spending cut.
The question is only seeking an understanding of the initial reduction in overal spending in the economy rather than the fully exhausted multiplied expansion of national income that will result.
It is clear that the tax increase will have two effects: (a) some initial spending contraction; and (b) it decreases the value of the multiplier, other things equal.
We are only interested in the first effect rather than the total effect.
But I will give you some insight also into what the two components of the tax result might imply overall when compared to cut in government spending.
To give you a concrete example which will consolidate the understanding of what happens, imagine that the marginal propensity to consume out of disposable income is 0.8 and there is only one tax rate set at 0.20.
So for every extra dollar that the economy produces the government taxes 20 cents leaving 80 cents in disposable income.
In turn, households then consume 0.8 of this 80 cents which means an injection of 64 cents goes into aggregate demand which them multiplies as the initial spending creates income which, in turn, generates more spending and so on.
Government spending cut
An cut in government spending (say of $1,000) is what we call an exogenous spending cut and reduces aggregate demand by that amount immediately.
Say it takes the form of a cut in procurement for books to school libraries.
The firm that supplies the books will experience reduced sales and will decrease book by $1,000. As a result incomes of the productive factors fall by $1000.
So the initial fall in aggregate demand is $1,000.
This initial decline in national output and income then induces further declines in consumption spending by 64 cents in the dollar so in Period 2, aggregate demand falls by $640.
Output and income decline by the same amount to meet this fall in spending.
In Period 3, aggregate demand falls again by 0.8 x $640 and so on.
The induced spending decrease gets smaller and smaller.
Tax rate increase
The contraction coming from a tax rate increase does not directly impact on the spending stream in the same way as the cut in government spending.
First, imagine the government worked out a tax rate rise that would decrease its initial fiscal deficit by the same amount as would have been the case if it had decreased government spending (so in our example, $1,000).
In other words, disposable income at each level of GDP initially falls by $1,000. What happens next?
Some of the loss in disposable income is accounted for by reduced saving (20 cents in each dollar that disposable income falls). So the immediate spending loss is $800 rather than $1,000.
The other $200 is accounted for by decreased overall saving.
What happens next depends on the parameters of the macroeconomic system.
The multiplied fall in national income may be higher or lower depending on these parameters.
But it will never be the case that an initial fiscal equivalent tax rise will be more contractionary than a government spending cut.
You may wish to read the following blog posts for more information:
That is enough for today!
(c) Copyright 2022 William Mitchell. All Rights Reserved.
That is enough for today!
(c) Copyright 2022 William Mitchell. All Rights Reserved.
This Post Has 0 Comments