The Weekend Quiz – February 6-7, 2016 – answers and discussion

February 6, 2016
bill
Saturday quiz
0 Comments

Here are the answers with discussion for yesterday’s quiz. The information provided should help you work out why you missed a question or three! If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of modern monetary theory (MMT) and its application to macroeconomic thinking. Comments as usual welcome, especially if I have made an error.

Question 1:

If a nation records an external balance (net exports equal zero) and the government runs a balanced fiscal position then we know that private domestic sector spending will be equal to its overall income and household saving will be zero.

The answer is False.

This is a question about sectoral balances.

To refresh your memory the balances are derived as follows. The basic income-expenditure model in macroeconomics can be viewed in (at least) two ways: (a) from the perspective of the sources of spending; and (b) from the perspective of the uses of the income produced. Bringing these two perspectives (of the same thing) together generates the sectoral balances.

From the sources perspective we write:

GDP = C + I + G + (X – M)

which says that total national income (GDP) is the sum of total final consumption spending (C), total private investment (I), total government spending (G) and net exports (X – M).

Expression (1) tells us that total income in the economy per period will be exactly equal to total spending from all sources of expenditure.

We also have to acknowledge that financial balances of the sectors are impacted by net government taxes (T) which includes all taxes and transfer and interest payments (the latter are not counted independently in the expenditure Expression (1)).

Further, as noted above the trade account is only one aspect of the financial flows between the domestic economy and the external sector. we have to include net external income flows (FNI).

Adding in the net external income flows (FNI) to Expression (2) for GDP we get the familiar gross national product or gross national income measure (GNP):

(2) GNP = C + I + G + (X – M) + FNI

To render this approach into the sectoral balances form, we subtract total taxes and transfers (T) from both sides of Expression (3) to get:

(3) GNP – T = C + I + G + (X – M) + FNI – T

Now we can collect the terms by arranging them according to the three sectoral balances:

(4) (GNP – C – T) – I = (G – T) + (X – M + FNI)

The the terms in Expression (4) are relatively easy to understand now.

The term (GNP – C – T) represents total income less the amount consumed less the amount paid to government in taxes (taking into account transfers coming the other way). In other words, it represents private domestic saving.

The left-hand side of Equation (4), (GNP – C – T) – I, thus is the overall saving of the private domestic sector, which is distinct from total household saving denoted by the term (GNP – C – T).

In other words, the left-hand side of Equation (4) is the private domestic financial balance and if it is positive then the sector is spending less than its total income and if it is negative the sector is spending more than it total income.

The term (G – T) is the government financial balance and is in deficit if government spending (G) is greater than government tax revenue minus transfers (T), and in surplus if the balance is negative.

Finally, the other right-hand side term (X – M + FNI) is the external financial balance, commonly known as the current account balance (CAD). It is in surplus if positive and deficit if negative.

In English we could say that:

The private financial balance equals the sum of the government financial balance plus the current account balance.

We can re-write Expression (6) in this way to get the sectoral balances equation:

(5) (S – I) = (G – T) + CAD

which is interpreted as meaning that government sector deficits (G – T > 0) and current account surpluses (CAD > 0) generate national income and net financial assets for the private domestic sector.

Conversely, government surpluses (G – T < 0) and current account deficits (CAD < 0) reduce national income and undermine the capacity of the private domestic sector to add financial assets.

Expression (5) can also be written as:

(6) [(S – I) – CAD] = (G – T)

where the term on the left-hand side [(S – I) – CAD] is the non-government sector financial balance and is of equal and opposite sign to the government financial balance.

This is the familiar MMT statement that a government sector deficit (surplus) is equal dollar-for-dollar to the non-government sector surplus (deficit).

The sectoral balances equation says that total private savings (S) minus private investment (I) has to equal the public deficit (spending, G minus taxes, T) plus net exports (exports (X) minus imports (M)) plus net income transfers.

All these relationships (equations) hold as a matter of accounting and not matters of opinion.

Thus, when an external deficit (X – M < 0) and public surplus (G – T < 0) coincide, there must be a private deficit. While private spending can persist for a time under these conditions using the net savings of the external sector, the private sector becomes increasingly indebted in the process.

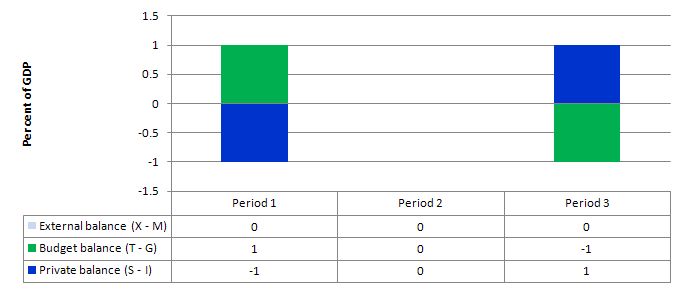
Consider the following graph which shows three situations where the external sector is in balance.

Period 1, the fiscal position is in surplus (T – G = 1) and the private domestic balance is in deficit (S – I = -1). With the external balance equal to 0, the general rule that the government surplus (deficit) equals the non-government deficit (surplus) applies to the government and the private domestic sector.

In Period 3, the fiscal position is in deficit (T – G = -1) and this provides some demand stimulus in the absence of any impact from the external sector, which allows the private domestic sector to save (S – I = 1).

Period 2, is the case in point and the sectoral balances show that if the external sector is in balance and the government is able to achieve a fiscal balance, then the private domestic sector must also be in balance.

The movements in income associated with the spending and revenue patterns will ensure these balances arise.

The problem is that if the private domestic sector desires to save overall then this outcome will be unstable.

So under the conditions of the question, the private domestic sector cannot save overall, even if the household sector was saving part of its disposable income. The government would be undermining any desire to save by not providing the fiscal stimulus necessary to increase national output and income so that private households/firms could save.

The question statement would have been deemed true if I had left out the last phrase “and household saving will be zero”. If the external sector and the government sector are in exact balance then the private domestic sector also have to be in exact spending-income balance.

But within that spending-income balance, the household sector may be saving a portion of their disposable income at a level equal to the level of investment spending in the firm sector, giving an overall balance between income and spending for the private domestic sector as a whole.

You may wish to read the following blogs for more information:

Question 2:

The standard of living of workers falls if growth in real wages fails to keep pace with labour productivity growth.

The answer is False.

Under the conditions specified there are several things we can conclude:

Real wages are growing.
Labour productivity is growing faster.
The wage share is falling and Real Unit Labour Costs are falling.
The workers’ material standard of living is higher than if real wages growth was zero or worse.
That the rise in material living standards are less than would be indicated by the workers contribution to production.

To see why these points are so consider this.

The wage share in nominal GDP is expressed as the total wage bill as a percentage of nominal GDP. Economists differentiate between nominal GDP ($GDP), which is total output produced at market prices and real GDP (GDP), which is the actual physical equivalent of the nominal GDP. We will come back to that distinction soon.

To compute the wage share we need to consider total labour costs in production and the flow of production ($GDP) each period.

Employment (L) is a stock and is measured in persons (averaged over some period like a month or a quarter or a year.

The wage bill is a flow and is the product of total employment (L) and the average wage (w) prevailing at any point in time. Stocks (L) become flows if it is multiplied by a flow variable (W). So the wage bill is the total labour costs in production per period.

So the wage bill = W.L

The wage share is just the total labour costs expressed as a proportion of $GDP – (W.L)/$GDP in nominal terms, usually expressed as a
percentage. We can actually break this down further.

Labour productivity (LP) is the units of real GDP per person employed per period. Using the symbols already defined this can be written as:

LP = GDP/L

so it tells us what real output (GDP) each labour unit that is added to production produces on average.

We can also define another term that is regularly used in the media – the real wage – which is the purchasing power equivalent on the nominal wage that workers get paid each period. To compute the real wage we need to consider two variables: (a) the nominal wage (W) and the aggregate price level (P).

The real wage (w) tells us what volume of real goods and services the nominal wage (W) will be able to command and is obviously influenced by the level of W and the price level. For a given W, the lower is P the greater the purchasing power of the nominal wage and so the higher is the real wage (w).

We write the real wage (w) as W/P. So if W = 10 and P = 1, then the real wage (w) = 10 meaning that the current wage will buy 10 units of real output. If P rose to 2 then w = 5, meaning the real wage was now cut by one-half.

But if real wages are growing then the workers have greater purchasing power in real terms and are thus better off. So that is independent of what is happening to productivity.

Nominal GDP ($GDP) can be written as P.GDP, where the P values the real physical output.

Now if you put of these concepts together you get an interesting framework. To help you follow the logic here are the terms developed and be careful not to confuse $GDP (nominal) with GDP (real):

Wage share = (W.L)/$GDP
Nominal GDP: $GDP = P.GDP
Labour productivity: LP = GDP/L
Real wage: w = W/P

By substituting the expression for Nominal GDP into the wage share measure we get:

Wage share = (W.L)/P.GDP

In this area of economics, we often look for alternative way to write this expression – it maintains the equivalence (that is, obeys all the rules of algebra) but presents the expression (in this case the wage share) in a different “view”.
So we can write as an equivalent:

Wage share – (W/P).(L/GDP)

Now if you note that (L/GDP) is the inverse (reciprocal) of the labour productivity term (GDP/L). We can use another rule of algebra (reversing the invert and multiply rule) to rewrite this expression again in a more interpretable fashion.

So an equivalent but more convenient measure of the wage share is:

Wage share = (W/P)/(GDP/L) – that is, the real wage (W/P) divided by labour productivity (GDP/L).

If the growth in the real wage equals labour productivity growth the wage share is constant. The algebra is simple but we have done enough of that already.

Two other points to note. The wage share is also equivalent to the real unit labour cost (RULC) measures that Treasuries and central banks use to describe trends in costs within the economy. Please read my blog – Saturday Quiz – May 15, 2010 – answers and discussion – for more discussion on this point.

Now it becomes obvious that if the nominal wage (W) and the price level (P) are growing at the pace the real wage is constant. And if the real wage is growing at the same rate as labour productivity, then both terms in the wage share ratio are equal and so the wage share is constant.

The wage share was constant for a long time during the Post Second World period and this constancy was so marked that Kaldor (the Cambridge economist) termed it one of the great “stylised” facts. So real wages grew in line with
productivity growth which was the source of increasing living standards for workers.

The productivity growth provided the “room” in the distribution system for workers to enjoy a greater command over real production and thus higher living standards without threatening inflation.

Since the mid-1980s, the neo-liberal assault on workers’ rights (trade union attacks; deregulation; privatisation; persistently high unemployment) has seen this nexus between real wages and labour productivity growth broken. So while real wages have been stagnant or growing modestly, this growth has been dwarfed by labour productivity growth.

So the wage share has fallen in many nations operating under these conditions. Thus workers could have enjoyed much higher material living standards if they could have claimed more of the productivity growth (and kept the wage share constant).

The following blogs may be of further interest to you:

Question 3:

Rising private domestic saving overall signals the need for an expanding public deficit to avoid employment losses.

The answer is False.

The answer also relates to the sectoral balances framework outlined in detail above. When the private domestic sector decides to lift its saving ratio, we normally think of this in terms of households reducing consumption spending. However, it could also be evidenced by a drop in investment spending (building productive capacity).

The normal inventory-cycle view of what happens next goes like this. Output and employment are functions of aggregate spending. Firms form expectations of future aggregate demand and produce accordingly. They are uncertain about the actual demand that will be realised as the output emerges from the production process.

The first signal firms get that household consumption is falling is in the unintended build-up of inventories. That signals to firms that they were overly optimistic about the level of demand in that particular period.

Once this realisation becomes consolidated, that is, firms generally realise they have over-produced, output starts to fall. Firms layoff workers and the loss of income starts to multiply as those workers reduce their spending elsewhere.

At that point, the economy is heading for a recession. Interestingly, the attempts by households overall to increase their saving ratio may be thwarted because income losses cause loss of saving in aggregate – the is the Paradox of Thrift. While one household can easily increase its saving ratio through discipline, if all households try to do that then they will fail. This is an important statement about why macroeconomics is a separate field of study.

Typically, the only way to avoid these spiralling employment losses would be for an exogenous intervention to occur – in the form of an expanding public deficit. The fiscal position of the government would be heading towards, into or into a larger deficit depending on the starting position as a result of the automatic stabilisers anyway.

So an intuitive reasoning suggests that a demand gap opens and the only way to stop the economy from contracting with employment losses if it the government fills the spending gap by expanding net spending (its deficit).

However, this would ignore the movements in the third sector – there is also an external sector. It is possible that at the same time that the households are reducing their consumption as an attempt to lift the saving ratio, net exports boom. A net exports boom adds to aggregate demand (the spending injection via exports is greater than the spending leakage via imports).

So it is possible that the public fiscal balance could actually go towards surplus and the private domestic sector increase its saving ratio if net exports were strong enough.

The important point is that the three sectors add to demand in their own ways. Total GDP and employment are dependent on aggregate demand. Variations in aggregate demand thus cause variations in output (GDP), incomes and employment. But a variation in spending in one sector can be made up via offsetting changes in the other sectors.

The following blogs may be of further interest to you: