Saturday Quiz – September 19, 2015 – answers and discussion

Here are the answers with discussion for yesterday’s quiz. The information provided should help you understand the reasoning behind the answers. If you haven’t already done the Quiz from yesterday then have a go at it before you read the answers. I hope this helps you develop an understanding of Modern Monetary Theory (MMT) and its application to macroeconomic thinking. Comments as usual welcome, especially if I have made an error.

Question 1:

An external surplus is a necessary but not sufficient condition for a nation that wishes to grow during a period when the government is running a fiscal surplus and the private domestic sector is net saving.

The answer is True.

This is a question about the relative magnitude of the sectoral balances – the government fiscal balance, the external balance and the private domestic balance. The balances taken together always add to zero because they are derived as an accounting identity from the national accounts.

The balances reflect the underlying economic behaviour in each sector which is interdependent – given this is a macroeconomic system we are considering.

To refresh your memory the balances are derived as follows. The basic income-expenditure model in macroeconomics can be viewed in (at least) two ways: (a) from the perspective of the sources of spending; and (b) from the perspective of the uses of the income produced. Bringing these two perspectives (of the same thing) together generates the sectoral balances.

From the sources perspective we write:

GDP = C + I + G + (X – M)

which says that total national income (GDP) is the sum of total final consumption spending (C), total private investment (I), total government spending (G) and net exports (X – M).

From the uses perspective, national income (GDP) can be used for:

GDP = C + S + T

which says that GDP (income) ultimately comes back to households who consume (C), save (S) or pay taxes (T) with it once all the distributions are made.

Equating these two perspectives we get:

C + S + T = GDP = C + I + G + (X – M)

So after simplification (but obeying the equation) we get the sectoral balances view of the national accounts.

(I – S) + (G – T) + (X – M) = 0

That is the three balances have to sum to zero. The sectoral balances derived are:

• The private domestic balance (I – S) – positive if in deficit, negative if in surplus.
• The Budget Deficit (G – T) – negative if in surplus, positive if in deficit.
• The Current Account balance (X – M) – positive if in surplus, negative if in deficit.

These balances are usually expressed as a per cent of GDP but that doesn’t alter the accounting rules that they sum to zero, it just means the balance to GDP ratios sum to zero.

A simplification is to add (I – S) + (X – M) and call it the non-government sector. Then you get the basic result that the government balance equals exactly $-for-$ (absolutely or as a per cent of GDP) the non-government balance (the sum of the private domestic and external balances).

This is also a basic rule derived from the national accounts and has to apply at all times.

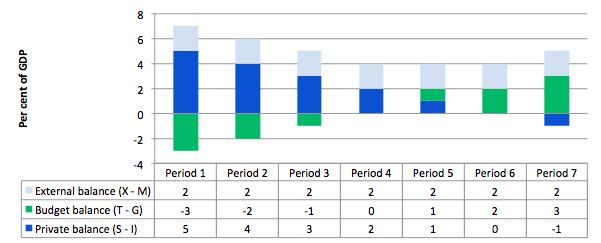
The following graph with accompanying data table lets you see the evolution of the balances expressed in terms of percent of GDP. In each period I just held the fiscal balance at a constant surplus (2 per cent of GDP) (green bars). This is is artificial because as economic activity changes the automatic stabilisers would lead to endogenous changes in the fiscal balance. But we will just assume there is no change for simplicity. It doesn’t violate the logic.

To aid interpretation remember that (I-S) > 0 means that the private domestic sector is spending more than they are earning; that (G-T) < 0 means that the government is running a surplus because T > G; and (X-M) < 0 means the external position is in deficit because imports are greater than exports.

If the nation is running an external surplus it means that the contribution to aggregate demand from the external sector is positive – that is net addition to spending which would increase output and national income.

The external surplus also means that foreigners are decreasing financial claims denominated in the local currency. Given that exports represent a real cost and imports a real benefit, the motivation for a nation running a net exports surplus (the exporting nation in this case) must be to accumulate financial claims (assets) denominated in the currency of the nation running the external deficit.

A fiscal surplus means the government is spending less than it is taking out of the economy via taxation which puts a drag on aggregate demand and constrains the ability of the economy to grow. So the question is what are the relative magnitudes of the external add and the fiscal subtract from income?

The following graph shows the range of options for a given external surplus (of 2 per cent of GDP).

In Periods 1 to 5, the private sector is saving because the public sector does not negate the overall contribution of the external sector to demand and hence growth. Clearly, the larger is the fiscal deficit the greater is the capacity of the private sector to save overall because the growth in income would be stronger.

In Periods 4 and 5, the fiscal balance moves from deficit to balance then surplus, yet the private sector can still net save. That is because the fiscal drag coming from the fiscal balance in Period 4 is zero and in Period 5 less than the aggregate demand add derived from the external sector.

In Periods 6 and 7, the private sector stops net saving because the fiscal drag coming from the fiscal surplus offsets (Period 6) and then overwhelms (Period 7) the aggregate demand add from the external sector.

The general rule when the economy runs an external surplus is that the private domestic sector will be able to net save if the fiscal surplus is less than the external surplus.

That is why the external surplus is necessary but not sufficient. It relies on the fiscal surplus being large enough for the private domestic sector to be able to net save.

The following blogs may be of further interest to you:

• Barnaby, better to walk before we run
• Stock-flow consistent macro models
• Norway and sectoral balances
• The OECD is at it again!
• Saturday Quiz – June 19, 2010 – answers and discussion

Question 2:

If central banks stopped paying a return to the private banks on the reserves they hold with the central bank then the private banks would have a greater incentive to advance credit to the private sector.

The answer is False.

The facts are as follows. First, central banks will always provided enough reserve balances to the commercial banks at a price it sets using a combination of overdraft/discounting facilities and open market operations.

Second, if the central bank didn’t provide the reserves necessary to match the growth in deposits in the commercial banking system then the payments system would grind to a halt and there would be significant hikes in the interbank rate of interest and a wedge between it and the policy (target) rate – meaning the central bank’s policy stance becomes compromised.

Third, any reserve requirements within this context while legally enforceable (via fines etc) do not constrain the commercial bank credit creation capacity. Central bank reserves (the accounts the commercial banks keep with the central bank) are not used to make loans. They only function to facilitate the payments system (apart from satisfying any reserve requirements).

Fourth, banks make loans to credit-worthy borrowers and these loans create deposits. If the commercial bank in question is unable to get the reserves necessary to meet the requirements from other sources (other banks) then the central bank has to provide them. But the process of gaining the necessary reserves is a separate and subsequent bank operation to the deposit creation (via the loan).

Fifth, if there were too many reserves in the system (relative to the banks’ desired levels to facilitate the payments system and the required reserves then competition in the interbank (overnight) market would drive the interest rate down. This competition would be driven by banks holding surplus reserves (to their requirements) trying to lend them overnight. The opposite would happen if there were too few reserves supplied by the central bank. Then the chase for overnight funds would drive rates up.

In both cases the central bank would lose control of its current policy rate as the divergence between it and the interbank rate widened. This divergence can snake between the rate that the central bank pays on excess reserves (this rate varies between countries and overtime but before the crisis was zero in Japan and the US) and the penalty rate that the central bank seeks for providing the commercial banks access to the overdraft/discount facility.

So the aim of the central bank is to issue just as many reserves that are required for the law and the banks’ own desires.

But banks do not lend reserves. They are used to facilitate the so-called payments system so that all transactions that are drawn on the various banks (cheques etc) clear at the end of each day. Clearly banks prefer to earn a return on reserves that it deems are in excess of its clearing house (payments system) requirements.

But in the absence of such a return being paid by the central bank the only consequence would be that the banks (overall) would have zero interest balances.

You might like to read this blog for further information:

• US federal reserve governor is part of the problem

Question 3:

A nation can only start to reduce its public debt to GDP ratio when it succeeds in running primary fiscal surpluses (that is, its spending net of interest payments is less than taxation revenue).

The answer is False.

While Modern Monetary Theory (MMT) places no particular importance in the public debt to GDP ratio for a sovereign government, given that insolvency is not an issue, the mainstream debate is dominated by the concept. The unnecessary practice of fiat currency-issuing governments of issuing public debt $-for-$ to match public net spending (deficits) ensures that the debt levels will always rise when there are deficits.

But the rising debt levels do not necessarily have to rise at the same rate as GDP grows. The question is about the debt ratio not the level of debt per se.

Rising deficits often are associated with declining economic activity (especially if there is no evidence of accelerating inflation) which suggests that the debt/GDP ratio may be rising because the denominator is also likely to be falling or rising below trend.

Further, historical experience tells us that when economic growth resumes after a major recession, during which the public debt ratio can rise sharply, the latter always declines again.

It is this endogenous nature of the ratio that suggests it is far more important to focus on the underlying economic problems which the public debt ratio just mirrors.

The mainstream framework for analysing the dynamics in public debt ratios starts with the concept of the government fiscal constraint (GBC). The GBC says that the fiscal deficit in year t is equal to the change in government debt over year t plus the change in high powered money over year t. So in mathematical terms it is written as:

which you can read in English as saying that Budget deficit = Government spending + Government interest payments – Tax receipts must equal (be “financed” by) a change in Bonds (B) and/or a change in high powered money (H). The triangle sign (delta) is just shorthand for the change in a variable.

However, this is merely an accounting statement. In a stock-flow consistent macroeconomics, this statement will always hold. That is, it has to be true if all the transactions between the government and non-government sector have been correctly added and subtracted.

For a sovereign government that issues its own currency, the previous equation is just an ex post accounting identity that has to be true by definition and has no real economic importance.

However, for nations such as Greece, which has ceded its currency sovereignty, the GBC becomes an financial constraints given that it has to fund its spending from taxation and/or bond issues.

A primary fiscal balance is the difference between government spending (excluding interest rate servicing) and taxation revenue.

The standard mainstream framework is usually expressed in terms of the ratio of debt to GDP rather than the level of debt per se. Even so-called progressives (deficit-doves) use this framework as if it applies to all governments.

The following equation captures the approach:

So the change in the debt ratio is the sum of two terms on the right-hand side: (a) the difference between the real interest rate (r) and the GDP growth rate (g) times the initial debt ratio; and (b) the ratio of the primary deficit (G-T) to GDP.

The real interest rate is the difference between the nominal interest rate and the inflation rate.

This standard mainstream framework is used to highlight the dangers of running deficits. But even progressives (not me) use it in a perverse way to justify deficits in a downturn balanced by surpluses in the upturn.

Many mainstream economists and a fair number of so-called progressive economists say that governments should as some point in the business cycle run primary surpluses (taxation revenue in excess of non-interest government spending) to start reducing the debt ratio back to “safe” territory.

Almost all the media commentators that you read on this topic take it for granted that the only way to reduce the public debt ratio is to run primary surpluses. That is what the whole “credible exit strategy” rhetoric is about and what is driving the austerity push around the world at present.

So the question is whether continuous national governments deficits imply continuously rising public debt levels as a percentage of GDP and whether primary fiscal surpluses are required to reduce the public debt ratio.

While MMT advocates running fiscal deficits when they are necessary to fill a spending gap left by non-government saving, it also emphasises that a government running a deficit can also reduce the debt ratio if it stimulates growth.

The standard formula above can easily demonstrate that a nation running a primary deficit can reduce its public debt ratio over time.

Furthermore, depending on contributions from the external sector, a nation running a deficit will more likely create the conditions for a reduction in the public debt ratio than a nation that introduces an austerity plan aimed at running primary surpluses.

Here is why that is the case. A growing economy can absorb more debt and keep the debt ratio constant or falling. From the formula above, if the primary fiscal balance is zero, public debt increases at a rate r but the public debt ratio increases at r – g.

The orthodox economists use this analysis to argue that permanent deficits are bad because the financial markets will “penalise” a government living on debt. If the public debt ratio is “too high” (whatever that is or means), markets “lose faith” in the government.

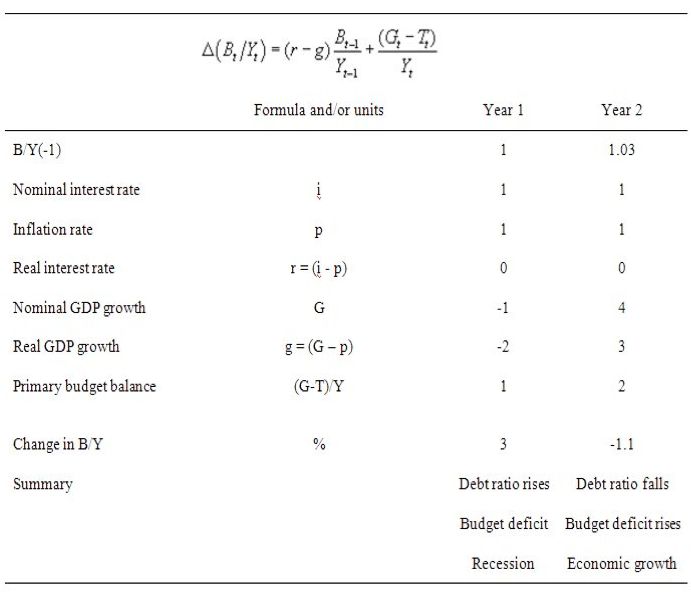
Consider the following Table which shows two years in the life of an economy.

It keeps things simple by assuming a public debt ratio at the start of the period of 100 per cent (so B/Y(-1) = 1).

Assume that the real rate of interest is 0 (so the nominal interest rate equals the inflation rate) – not to dissimilar to the situation at present in many countries.

Assume that the rate of real GDP growth is minus 2 per cent (that is, the nation is in recession) and the automatic stabilisers push the primary fiscal balance into deficit equal to 1 per cent of GDP. As a consequence, the public debt ratio will rise by 3 per cent. So in Year 2, the debt ratio is 1.03 of GDP.

The government reacts to the recession in the correct manner and increases its discretionary net spending to take the deficit in Year 2 to 2 per cent of GDP (noting a positive number in this instance is a deficit).

The central bank maintains its zero interest rate policy and the inflation rate also remains at zero so the real interest rate doesn’t move.

The increasing deficit stimulates economic growth in Year 2 such that real GDP grows by 3 per cent. In this case the public debt ratio falls by 1 per cent.

So even with an increasing (or unchanged) deficit, real GDP growth can reduce the public debt ratio, which is what has happened many times in past history following economic slowdowns.

In other words, a government such as Greece does not have to run fiscal surpluses to bring its public debt ratio down. What it needs is growth and that is more likely to occur if it holds its nerve and runs deficits. The problem is that the membership of the EMU (lack of currency sovereignty) makes that difficult without ECB support.

The best way to reduce the public debt ratio is to stop issuing debt. A sovereign government doesn’t have to issue debt if the central bank is happy to keep its target interest rate at zero or pay interest on excess reserves.

The discussion also demonstrates why tightening monetary policy makes it harder for the government to reduce the public debt ratio – which, of-course, is one of the more subtle mainstream ways to force the government to run surpluses.

The following blog may be of further interest to you:

• Saturday Quiz – May 8, 2010 – answers and discussion