It’s Wednesday and I just finished a ‘Conversation’ with the Economics Society of Australia, where I talked about Modern Monetary Theory (MMT) and its application to current policy issues. Some of the questions were excellent and challenging to answer, which is the best way. You can view an edited version of the discussion below and…
Aggregate Demand Part 2
I am now using Friday’s blog space to provide draft versions of the Modern Monetary Theory textbook that I am writing with my colleague and friend Randy Wray. We expect to complete the text by the end of this year. Comments are always welcome. Remember this is a textbook aimed at undergraduate students and so the writing will be different from my usual blog free-for-all. Note also that the text I post is just the work I am doing by way of the first draft so the material posted will not represent the complete text. Further it will change once the two of us have edited it.
This continues the Chapter on Aggregate Demand and Output and Income generation.
Aggregate Demand
In Chapter 7, we learned that firms build a stock of productive capital through investment in order to produce goods and services to satisfy demand. One the capital stock in in place, firms will respond to increases in spending for the goods and services they supply by increasing output up to the productive limits of their capital and the available labour and other inputs. Beyond full capacity, they can only increase prices when increased spending occurs.
Aggregate demand is the total purchases by households, firms, government and foreigners (rest of the world) on goods and services produced by domestic and foreign firms. The volume of real output supplied to the economy is determined by aggregate demand subject to there being idle productive capacity.
The causal chain is SPENDING => OUTPUT => EMPLOYMENT => UNEMPLOYMENT (for a given labour force) and CAPACITY UTILISATION
As long as their is productive capacity available, this causal chain will be active.
The task of this Section is to examine in more detail the components of total spending and explain how they interact to determine total output (GDP) and national income.
For this purpose we are assuming that potential output is fixed for the period we are analysing. We will show that investment builds new capital and population growth adds new workers to the economy both of which expand potential output over time. In Chapter 12 we will consider economic growth and examine how aggregate demand and potential output can move over time.
We will learn that even if aggregate spending might currently be sufficient to maintain the full employment of available labour, growth in the supply-side of the economy – that is, potential output – will require ever more growth in aggregate demand in the future. In effect, with on-going investment, the economy chases itself to maintain full employment.
But for now we assume that potential output is fixed and that firms supply up to that potential according to the aggregate spending that prevails in any period.
In Chapter 5 we introduced the National Accounting framework, which we expanded in Chapter 6 Sectoral Accounting and in Chapter 7 Introduction to Effective Demand. In that framework, we defined the major components of aggregate spending that the national statisticial agencies measure when compiling the national accounting estimates of GDP.
The convention is to divide spending into household consumption; business investment by firms; government spending; export spending by foreigners and import spending by domestic residents.
In the remainder of this Chapter we build a model of aggregate spending and income determination by progressively adding these components. In doing so we will develop an understanding of the behaviour of each of these sectors and how that behaviour interacts. We first consider private or household consumption.
Private Consumption Expenditure
In Chapter 3 we discussed the role of government and the concept of money and learned that in a fiat-currency system the government issues the currency which the non-government sector uses. This gave us a very advanced understanding of how financial assets can enter the economy – with government at the centre of the stage.
In this Section we keep that understanding in the back of our mind but focus on private consumption spending on goods and services.
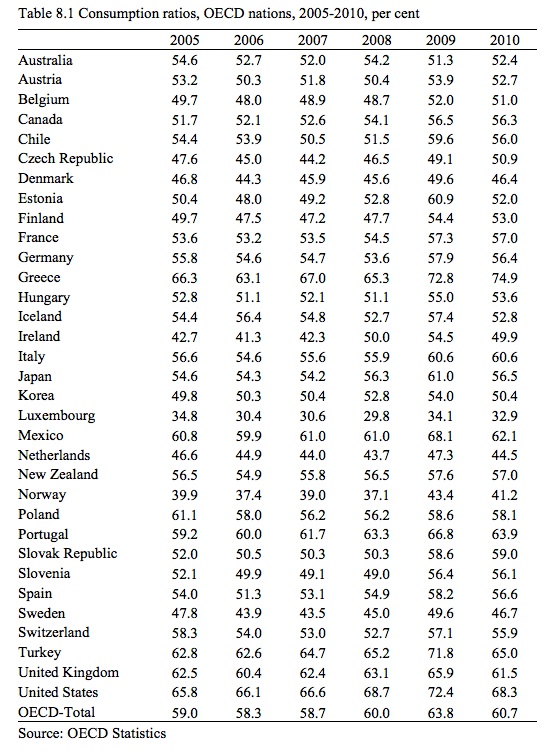
Table 8.1 shows the proportion of Private consumption expenditure in GDP for the OECD nations less Israel. While there are notable exceptions, most nations are around the OECD average of 60.7 per cent. Private consumption expenditure is the largest component of total spending on GDP. The ratio is also relatively stable over time.
Note that this Table shows the ratio of consumption to total GDP. In the next section we will relate total consumption spending to what economists call disposable income, which is total income (Y) less the amount that governments take out in the form of taxes (T).
What determines private consumption expenditure?
The most elementary theory of private consumption (C) says that it is a stable proportional function of disposable national income (Yd). We thus define the consumption function as:
(8.2) C = cYd
where c is the marginal propensity to consume (MPC) or the fraction of every dollar of disposable income consumed. The MPC is posited to be between 0 and 1. If, for example, c = 0.8 then for every extra dollar of dispoable income that the economy generates consumption would rise by 80 cents.
It is important to understand that the MPC in this model is an aggregate which is an average of all the individual household consumption propensities. Lower income households tend to have MPC values close to 1 whereas the higher income households have much lower than average consumption propensities.
This arises because lower income families have less in consumption in absolute terms and find it harder to meet their necessary expenditure to maintain basic survival given their income levels. Higher income earners not only consume more in absolute terms but have much more income free after they have purchased all the essentials to maintain life.
As we will learn later in this chapter, the distribution of income is an important consideration when seeking to understand changes in aggregate demand. For example, a change in tax policy that increased disposable income for low-income consumers would have a greater positive impact on final consumption that a tax cut aimed at giving high-income earners the same absolute increase in disposable income.
We define disposable income as:
(8.3) Yd = Y – T
The difference between consumption (C) and disposable income is private saving (S). We can write that as:
(8.4) S = Yd – C = Y – T – C
Saving at the macroeconomic level is thus the residual that is left over from disposable income after households have made their consumption choices and total income has been generated.
If the MPC or c is the proportion of disposable income that is consumed then we can define a related concept – the marginal propensity to save (s) which is just the 1 minus the marginal propensity to consume (c).
Equation (8.2) can be substituted in the saving equation (8.4) to get what we call the saving function:
(8.5) S = Yd – C = Yd – cYd = (1 – c )Yd
The saving function tells us that total saving in the economy is a function of total disposable income. The higher is the level of GDP for a given tax regime, the higher will saving and consumption will be.
The marginal propensity to save, s = (1-c). Thus, if the MPC was 0.8 we know that s = 0.2, which means that for every extra dollar of disposable income generated in the economy 20 cents will be saved. If the MPC fell, then that proportion saved would rise.
The most elementary theory of private consumption (C) says that it is a stable proportional function of disposable national income (Yd). We thus define the consumption function as:
(8.2) C = cYd
where c is the marginal propensity to consume (MPC) or the fraction of every dollar of disposable income consumed. The MPC is posited to be between 0 and 1. If, for example, c = 0.8 then for every extra dollar of dispoable income that the economy generates consumption would rise by 80 cents.
It is important to understand that the MPC in this model is an aggregate which is an average of all the individual household consumption propensities. Lower income households tend to have MPC values close to 1 whereas the higher income households have much lower than average consumption propensities.
This arises because lower income families have less in consumption in absolute terms and find it harder to meet their necessary expenditure to maintain basic survival given their income levels. Higher income earners not only consume more in absolute terms but have much more income free after they have purchased all the essentials to maintain life.
As we will learn later in this chapter, the distribution of income is an important consideration when seeking to understand changes in aggregate demand. For example, a change in tax policy that increased disposable income for low-income consumers would have a greater positive impact on final consumption that a tax cut aimed at giving high-income earners the same absolute increase in disposable income.
We define disposable income as:
(8.3) Yd = Y – T
The difference between consumption (C) and disposable income is private saving (S). We can write that as:
(8.4) S = Yd – C = Y – T – C
Saving at the macroeconomic level is thus the residual that is left over from disposable income after households have made their consumption choices and total income has been generated.
If the MPC or c is the proportion of disposable income that is consumed then we can define a related concept – the marginal propensity to save (s) which is just the reciprocal of c.
Equation (8.2) can be substituted in the saving equation (8.4) to get what we call the saving function:
(8.5) S = Yd – C = Yd – cYd = (1 – c )Yd
The saving function tells us that total saving in the economy is a function of total disposable income. The higher is the level of GDP for a given tax regime, the higher will saving and consumption will be.
The marginal propensity to save, s = (1-c). Thus, if the MPC was 0.8 we know that s = 0.2, which means that for every extra dollar of disposable income generated in the economy 20 cents will be saved. If the MPC fell, then that proportion saved would rise.
Often we generalise Equation (8.2) in the following way:
(8.2a) C = C0 + cYd
where C0 is considered some base level of consumption which is independent of disposable income. It also means the saving function (8.5) woud be S = -C0 + (1 – c)Yd.
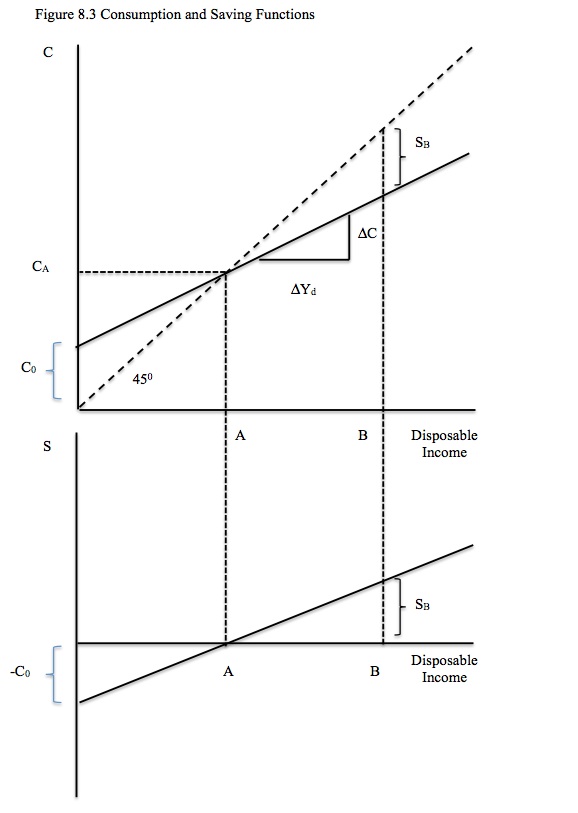
We express these relationships graphically in Figure 8.3 which shows the consumption function in the upper figure and the saving function in the lower figure. We have drawn the consumption function in the 450 diagram except the horizontal axis in this case is disposable income and the vertical axis measures total private consumption spending (C) in the upper figure and total private saving (S) in the lower figure.
All points on the 450 line measure points where all consumption equals disposable income and there is no saving.
The vertical intercepts are positive for the consumption function if C0 > 0 (that case is shown), which means the vertical intercept for the saving function is negative (-C0).
Both functions are upward sloping because we have postulated that consumption and the residual saving are positive functions of disposable income. In Chapter 4 Methods, Tools and Techniques we learned how to derive a slope graphically. We said that the slope of a line is the ratio RISE over RUN. Rise in this case is the change in consumption spending (ΔC) and run is the change in disposable income (ΔYd) and we have drawn a little triangle underneath the consumption function to show that.
In fact, ΔC = cΔYd and RISE over RUN = ΔC/ΔYd or cΔYd/ΔYd = c. So the slope of the consumption function in graphical terms is the MPC, which makes sense because we defined it as the change in consumption for a given change in disposable income.
Consider disposable income level A. At that point, consumption crosses the 450 line, which means it is equal to disposable income. At that point there is no aggregate saving. All disposable income is consumed. Disposable income levels between 0 and Point A signify that aggregate consumption is greater than disposable income which we refer to as dis-saving. If you look at the saving function you will see that it lies below the horizontal axis up to Point A and is thus negative.
After point A, consumption is less than disposable income and saving becomes positive. So at Point B, Saving is measured as the gap between the 450 line and the consumption function (or simply from the specific saving function given).
You should be able to work out what would happen if the MPC (c) increased? The result would be an increase in the slope of the consumption function such that at every level of disposable income, total consumption would be higher.
Introducing government spending and taxation
To develop a deeper understanding of this simple economy we introduce government spending (G) and a proportional tax rate (t) in order to develop the concept of the expenditure multiplier.
Governments purchase a range of goods and services from the non-government sector. Some of the purchases are for consumption goods and services, which provide benefits over a single period (say a year), while other spending is categorised as public investment or public capital formation. The latter category of spending generates the valuable public infrastucture that enhances the welfare and profitability of the non-government sector.
Table 8.2 shows the proprtion of government spending in total GDP.
[NOTE – a brief descriptive section follows]
[NOTE: TABLE HERE WILL SHOW THE RATIO OF GOVERNMENT SPENDING TO TOTAL NATIONAL INCOME – WE ARE STILL TO DECIDE WHETHER TO CREATE ONE TABLE SHOWING ALL THE COMPONENTS OF THE NATIONAL ACCOUNTS FOR ONE YEAR RATHER THAN SEPARATE TABLES FOR EACH COMPONENT ACROSS A NUMBER OF YEARS AS IN TABLE 8.1]
In Chapter 13, we will learn that government spending overall is determined by two broad forces. First, the policy decisions that the government takes in setting its fiscal policy. Second, the state of the overall business cycle. For exampe, when the economy is performing badly, government spending will increase as a result of welfare payments even without any explicit change in government policy. The opposite will be the case when the economy is growing strongly and unemployment is falling.
We call these effects cyclical because they vary with the state of the economic cycle. We will consider them in more detail in Chapter 13 when we analyse budgets.
For the purposes of the following discussion, we will assume away these cyclical effects and consider government spending (G) to be given by the policy choice of the government and thus is exogenous, using the terminology we explained in Chapter 4.
The tax policy which sets the tax rate (t) shows that total tax revenue (T) is given as:
(8.6) T = tY
where t is the marginal tax rate.
Assume that the proportional tax rate (t) is 0.20. This means that for every dollar of national income generated the government takes 20 cents out in the form of taxation. The remaining 80 cents in left over as disposable income.
We consider taxation to be a “leakage” from the expenditure system because it is income that does not become spending.
Given that taxes are taken out of total income (Y), disposable income can be written as:
(8.7) Yd = Y – T = Y – tY – (1-t)Y
In our specific example, this would be written as Yd = (1 – 0.2)Y = 0.8Y. After substituting the expression for disposable income into the consumption function equation (8.2a) we get:
(8.8) C = C0 + cYd = C0 + c(1 – t)Y
Aggregate spending is the sum of consumption spending and (C) and government spending (G) so that GDP (Y) is given as:
(8.9) Y = C + G
Which we can write as:
Y = C0 + c(1 – t)Y + G
This simplifies to:
Y – c(1 – t)Y = C0 + G
Y(1 – c(1 – t)) = = C0 + G
Which generates what we call the equilibrium national income equation:
(8.11) Y = 1/(1 – c(1 – t))[C0 + G]
You will notice that the equilibrium income that results from the sum of consumption and government spending is the product of two exogenous spending components, C0 + G and the coefficient 1/(1 – c(1 – t)), which we call the expenditure multiplier.
We sometimes refer to the exogenous spending components as autonomous spending because they do not depend on national income – they are given.
The expenditure multiplier tells us how much national income (Y) will change for a given change in autonomous spending
The expenditure multiplier is a ratio involving the marginal propensity to consume (c) and the marginal tax rate (t). By inspection we can see that the higher is the MPC and the lower is the tax rate the larger will be the expenditure multiplier. The task now is to explain why that is the case in terms of the economic processes involved.
[NOTE: the next part is an explication of the expenditure multiplier – followed by the introduction of private investment and the external sector. And Appendix will also provide more complex functional forms to expand the intermediate student’s understanding. Remember that the book is aiming to span both the first-year and second-year of an undergraduate curriculum]
Saturday Quiz
The Saturday Quiz will be back again tomorrow. For all those smarties out there who are complaining it is too easy think about when you found it to be too hard.
That is enough for today!
(c) Copyright 2012 Bill Mitchell. All Rights Reserved.
This may be a foolish question but here goes. I am not quite sure how to read the following causal function or causal chain;
SPENDING => OUTPUT => EMPLOYMENT => UNEMPLOYMENT (for a given labour force) and CAPACITY UTILISATION.
If I literally substitute the word causes for the symbol “=>” I get;
SPENDING causes OUTPUT causes EMPLOYMENT causes UNEMPLOYMENT (for a given labour force) and CAPACITY UTILISATION.
I can’t really make heads or tails of the claim in this form. Maybe I should try;
Changes in SPENDING lead to changes in OUTPUT which lead to changes in EMPLOYMENT which lead to changes in UNEMPLOYMENT (for a given labour force) and CAPACITY UTILISATION.
Is this closer to the mark?
Just to be completely pedantic, I am sure you won’t be using the makeshift symbol “=>” when the book is typeset so the (admittedly nonsensical) confusion with “equal to or greater than” could never occur.
Some other (probably equally foolish) issues are;
I am a little concerned about modelling a straightforward, simplified causal chain like this for a system (the economy) which I strongly assume has feedback loops. I can see and agree with the point if deficit spending (creating government fiat money) primes the pump so to speak by increasing aggregate demand which prompts firms to utilise spare capacity and hire from the pool of unemployed (idle productive capacity being assumed as stated.)
I can’t actually see how to bring in the fact that the economy is also a feedback loop system in at least some ways. Do you further develop the model so that it moves forward in time and feedbacks are now modelled? For example, what happens if and when full capacity utilisation is reached? What happens when particular limits on full across-the-board capacity utilisation are hit? What if private capital becomes scarce first? What if full labour utilisation is reached first? What if transport infrastructure inadequacies are exposed before full capacity utilisation is reached? What if material or energetic resource shortages become apparent before full capacity utilisation is reached?
In particular, is it possible to ignore possible material or energetic resource shortages as we reach the earth’s limits to growth. Some of these limits have been reached now. Peak oil production is a clear example. And because of complex and compounding issues related to all energetic resources and their relation to GDP it may be that we are close to what will become an enduring peak energy plateau and this may enforce a “GDP” plateau at least in physical goods and physical infrastructure.
Are we not precisely at the geophysical-economic-historical juncture where these issues cannot be ignored?
Bill, I don’t think Ikonoclast’s concerns about notation are ‘silly’. One way of incorporating feedback loops and getting around problems depicting functional relationships would be to utilize an approach employed by Judea Pearl with some success in his Causality (both 1st & 2nd eds).
Symbolic logical considerations can illustrate clearly that f(g(x)) is not generally the same as g(f(x)), which some diagrammatic conventions render difficult to differentiate, especially egregious in a causal context.
A related issue is the matter of the consideration of evidence, an excellent account of which can be found in Analysis of Evidence by Anderson, Schum, and Twining (2nd ed, 2003). Not all the discussion is geared to legal considerations. They introduce a chart method for assessing evidential issues and hos they relate to one another invented by the jurist John Henry Wigmore around 1913 in Principle of Judicial Proof. This method is used with a good degree of success, but becomes quite complicated (and difficult), in Kadane & Schum, A Probabilistic Analysis of the Sacco and Vanzetti Evidence (1996). The treatment in Anderson, Schum, and Twining is much less complex and consequently easier to follow.
One you get past simple accounting algebra, where notational matters are trivial (mostly because solved), then notation can mean the difference between easily and not easily comprehended.
Choice of appropriate notation may be as important as the choice of publisher. Your former book is still over $100 is the US and £80 in the UK.
This is supposed to be a text book for students. It is not the be all and end all of economic literature.
Keep it simple enough so as it doesn’t scare students away and yet don’t dumb it down to the point whereby it fails to describe real world results.
I think Bill has the balance about right.
Bill, I think we should it explain in another way, but I don´t know, if you do it in another chapter:
Saving of the households at the macroeconomic level is limited. If there is no investment in new capital, there is no real saving. Without government the GDP must be the disposable income at point A and equal to the consumption. After introducing government and taxation, the GDP will increase. If the government spending G is only the taxation T, then the GDP is:
Y = A + T
The multiplier of the government spending out of taxation is 1. But if the government spending G is higher than taxation and the marginal propensity to consume is 0.8 you get 5 Dollar higher national income out of 1 Dollar higher government spending, the multiplier is 5. The GDP is increasing:
Y = A + 4x(G – T) + G
The saving function tells us that GDP and disposable income in the economy is a function of possible saving as long as there is productive capacity available.
“firms will respond to increases in spending for the goods and services they supply by increasing output up to the productive limits of their capital and the available labour and other inputs. Beyond full capacity, they can only increase prices when increased spending occurs.”
Why wouldn’t companies raise prices? Why would they automatically respond by increasing output?
“Why wouldn’t companies raise prices? Why would they automatically respond by increasing output?”
Defence of market share.
If you have companies operating in a competitive environment then you end up with a game of chicken regarding price rises. If you put your prices up to defray demand then you lose market share immediately to your competitors that have not put their prices up. However if you work harder and become more productive to service that extra demand at the current price then you get your share of the increase in demand – forcing your competitors to do the same.
Arguably if prices rise to defray a demand increase the competition authorities should be working out where the cartel/oligopoly is.
Over the financial crisis there has been a drop of in productivity across the western economies that classical economists struggle to explain. MMT, because it sees demand as variable from supply, would explain that as a build up of suppressed capacity. Firms have more labour and equipment than they strictly need for the current demand levels – hence why it looks like the productivity levels have dropped. Again that is against classical economics but fits perfectly with the way MMT explains the way the firm works – you don’t get rid of your skilled labour and equipment for a competitor to pick up in a fire sale unless you’re forced to.
And firms in a competitive area that know they have capacity to expand demand and will know that their competitors almost certainly have excess capacity to service any increase in demand. So the first response will be to quantity expand rather than price expand.
The ‘quantity expands first’ is a key tenet of the MMT approach. But it is a bit of an article of faith. There is some evidence to support the logic behind the belief though, but I will say it is far from conclusive or overwhelming. I would like to see more econometric support for the notion.
“Over the financial crisis there has been a drop of in productivity across the western economies that classical economists struggle to explain.”
An economist has argued this:
“On the supply-side, since the financial constraints have landed the economy with a smaller addition to its stock of capital than it would otherwise have had, it can only count on a smaller increase in the productivity of labour.”
“”On the supply-side, since the financial constraints have landed the economy with a smaller addition to its stock of capital than it would otherwise have had, it can only count on a smaller increase in the productivity of labour.”
I find the hoarding of labour more convincing. The divergence of the US – which had severe financial constraints but has gone for the increased unemployment approach due to their labour market design – suggests that is a labour function.
“Defence of market share”
So how do we explain the fact that businesses actually DO raise prices?
Possibly because perfect competition does not exist?
Also, businesses may view government stimulus as merely temporary, and so may opt to raise prices rather than expand output (i.e. take on more employees and increase investment). They are only likely to expand output and productive capacity if they think demand wil be sustained and sustainable. Which is not how many businesses see government stimulus.
“Also, businesses may view government stimulus as merely temporary, and so may opt to raise prices rather than expand output ”
They would have to do that en-mass, which would be cartel behaviour. It only take one to take an alternative view and suddenly the other competitors are playing catch up trying to defend their market share.
So to raise prices completely requires everybody to come to the same conclusion that is the only hope, whereas you can raise quantity independent of your competitors. Hence the latter has less resistance as an approach.
In fact in my experience businesses are more likely to raise prices in response to a general *drop* in demand in an attempt to stabilise their position.
Half the problem with economics is predicting the actions of the crowd. It’s like trying to predict the movements of a shoal of fish or a flock of geese. There is a general movement of the group, but individual movements within that group may be completely at odds to the general group movement.
Late entry, sorry. There are some hard to find typos in the text. If you don’t want them, send them back to me. Here they are, plus my corrections in brackets:
On(C)e the capital stock in in place,
As long as their (there) is productive capacity available,
that (than) a tax cut aimed at giving high-income earners the same absolute increase in disposable income.
then that (the) proportion saved would rise.
The vertical intercepts are (intercept is) positive for the consumption function
The remaining 80 cents in (is) left over as disposable income.
Notwithstanding the typos, the text should be just right for students.