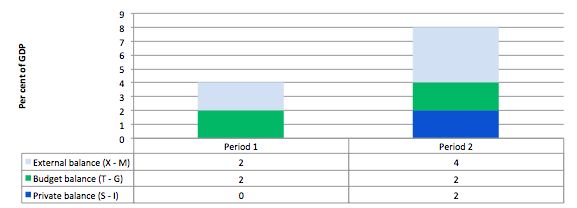
Start from a situation where the external surplus is the equivalent of 2 per cent of GDP and the government fiscal surplus surplus is 2 per cent. If the government fiscal balance stays constant as a per cent of GDP and the external surplus rises to the equivalent of 4 per cent of GDP, then you can conclude that national income also rises and the private surplus moves from 0 per cent of GDP to 2 per cent of GDP.
Answer: True
The answer is True.
This is a question about the sectoral balances and their link to national income dynamics via the expenditure system.
To refresh your memory the sectoral balances are derived as follows. The basic income-expenditure model in macroeconomics can be viewed in (at least) two ways: (a) from the perspective of the sources of spending; and (b) from the perspective of the uses of the income produced. Bringing these two perspectives (of the same thing) together generates the sectoral balances.
From the sources perspective we write:
GDP = C + I + G + (X - M)
which says that total national income (GDP) is the sum of total final consumption spending (C), total private investment (I), total government spending (G) and net exports (X - M).
Expression (1) tells us that total income in the economy per period will be exactly equal to total spending from all sources of expenditure.
We also have to acknowledge that financial balances of the sectors are impacted by net government taxes (T) which includes all taxes and transfer and interest payments (the latter are not counted independently in the expenditure Expression (1)).
Further, as noted above the trade account is only one aspect of the financial flows between the domestic economy and the external sector. we have to include net external income flows (FNI).
Adding in the net external income flows (FNI) to Expression (2) for GDP we get the familiar gross national product or gross national income measure (GNP):
(2) GNP = C + I + G + (X - M) + FNI
To render this approach into the sectoral balances form, we subtract total taxes and transfers (T) from both sides of Expression (3) to get:
(3) GNP - T = C + I + G + (X - M) + FNI - T
Now we can collect the terms by arranging them according to the three sectoral balances:
(4) (GNP - C - T) - I = (G - T) + (X - M + FNI)
The the terms in Expression (4) are relatively easy to understand now.
The term (GNP - C - T) represents total income less the amount consumed less the amount paid to government in taxes (taking into account transfers coming the other way). In other words, it represents private domestic saving.
The left-hand side of Equation (4), (GNP - C - T) - I, thus is the overall saving of the private domestic sector, which is distinct from total household saving denoted by the term (GNP - C - T).
In other words, the left-hand side of Equation (4) is the private domestic financial balance and if it is positive then the sector is spending less than its total income and if it is negative the sector is spending more than it total income.
The term (G - T) is the government financial balance and is in deficit if government spending (G) is greater than government tax revenue minus transfers (T), and in surplus if the balance is negative.
Finally, the other right-hand side term (X - M + FNI) is the external financial balance, commonly known as the current account balance (CAD). It is in surplus if positive and deficit if negative.
In English we could say that:
The private financial balance equals the sum of the government financial balance plus the current account balance.
We can re-write Expression (6) in this way to get the sectoral balances equation:
(5) (S - I) = (G - T) + CAD
which is interpreted as meaning that government sector deficits (G - T > 0) and current account surpluses (CAD > 0) generate national income and net financial assets for the private domestic sector.
Conversely, government surpluses (G - T < 0) and current account deficits (CAD < 0) reduce national income and undermine the capacity of the private domestic sector to add financial assets.
Expression (5) can also be written as:
(6) [(S - I) - CAD] = (G - T)
where the term on the left-hand side [(S - I) - CAD] is the non-government sector financial balance and is of equal and opposite sign to the government financial balance.
This is the familiar MMT statement that a government sector deficit (surplus) is equal dollar-for-dollar to the non-government sector surplus (deficit).
The sectoral balances equation says that total private savings (S) minus private investment (I) has to equal the public deficit (spending, G minus taxes, T) plus net exports (exports (X) minus imports (M)) plus net income transfers.
All these relationships (equations) hold as a matter of accounting and not matters of opinion.
Consider the following graph and accompanying table which depicts two periods outlined in the question.
In Period 1, with an external surplus of 2 per cent of GDP and a fiscal surplus of 2 per cent of GDP the private domestic balance is zero. The demand injection from the external sector is exactly offset by the demand drain (the fiscal drag) coming from the fiscal balance and so the private sector can neither net save or spend more than they earn.
In Period 2, with the external sector adding more to demand now - surplus equal to 4 per cent of GDP and the fiscal balance unchanged (this is stylised - in the real world the fiscal balance will certainly change), there is a stimulus to spending and national income would rise.
The rising national income also provides the capacity for the private sector to save overall and so they can now save 2 per cent of GDP.
The fiscal drag is overwhelmed by the rising net exports.
This is a highly stylised example and you could tell a myriad of stories that would be different in description but none that could alter the basic point.
If the drain on spending (from the public sector) is more than offset by an external demand injection, then GDP rises and the private sector overall saving increases.
If the drain on spending from the fiscal balance outweighs the external injections into the spending stream then GDP falls (or growth is reduced) and the overall private balance would fall into deficit.
You may wish to read the following blogs for more information: